Page 44 - Mathematics Class - XII

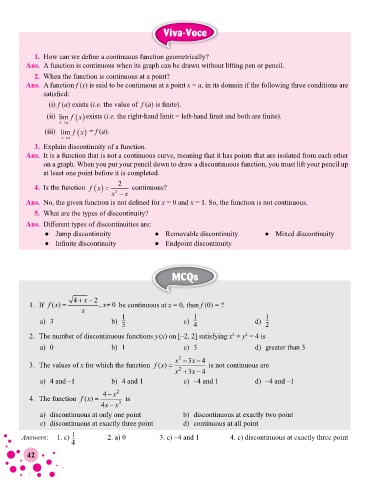
Viva-Voce

1. How can we define a continuous function geometrically?
Ans. A function is continuous when its graph can be drawn without lifting pen or pencil.

2. When the function is continuous at a point?
Ans. A function f (x) is said to be continuous at a point x = a, in its domain if the following three conditions are
satisfied:
(i) f (a) exists (i.e. the value of f (a) is finite).

(ii) lim fx exists (i.e. the right-hand limit = left-hand limit and both are finite).
x a
(iii) lim fx = f (a).
x a
3. Explain discontinuity of a function.
Ans. It is a function that is not a continuous curve, meaning that it has points that are isolated from each other
on a graph. When you put your pencil down to draw a discontinuous function, you must lift your pencil up
at least one point before it is completed.
2
4. Is the function fx continuous?
x x
2
Ans. No, the given function is not defined for x = 0 and x = 1. So, the function is not continuous.
5. What are the types of discontinuity?
Ans. Different types of discontinuities are:
● Jump discontinuity ● Removable discontinuity ● Mixed discontinuity
● Infinite discontinuity ● Endpoint discontinuity

MCQs

x 2
1. If fx() 4 x , 0 be continuous at x = 0, then f (0) = ?
x
a) 3 b) 1 c) 1 d) 1
4
3
2
2. The number of discontinuous functions y (x) on [–2, 2] satisfying x + y = 4 is
2
2
a) 0 b) 1 c) 3 d) greater than 3
2
3. The values of x for which the function fx() x 3 x 4 is not continuous are
2
x 3 x 4
a) 4 and –1 b) 4 and 1 c) –4 and 1 d) –4 and –1

4. The function fx() 4 x 2 is
4 xx 3
a) discontinuous at only one point b) discontinuous at exactly two point
c) discontinuous at exactly three point d) continuous at all point

1
Answers: 1. c) 2. a) 0 3. c) –4 and 1 4. c) discontinuous at exactly three point
4
42