Page 43 - Mathematics Class - XII

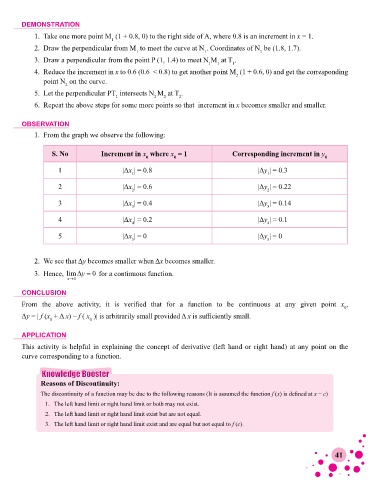
DEMONSTRATION
1. Take one more point M (1 + 0.8, 0) to the right side of A, where 0.8 is an increment in x = 1.
1
2. Draw the perpendicular from M to meet the curve at N . Coordinates of N be (1.8, 1.7).
1 1 1
3. Draw a perpendicular from the point P (1, 1.4) to meet N M at T .
1 1 1
4. Reduce the increment in x to 0.6 (0.6 < 0.8) to get another point M (1 + 0.6, 0) and get the corresponding
2
point N on the curve.
2
5. Let the perpendicular PT intersects N M at T .
1
2
2
2
6. Repeat the above steps for some more points so that increment in x becomes smaller and smaller.
OBSERVATION
1. From the graph we observe the following:

S. No Increment in x where x = 1 Corresponding increment in y 0
0
0
1 |Δx | = 0.8 |Δy | = 0.3
1
1
2 |Δx | = 0.6 |Δy | = 0.22
2 2
3 |Δx | = 0.4 |Δy | = 0.14
3 3
4 |Δx | = 0.2 |Δy | = 0.1
4 4

5 |Δx | = 0 |Δy | = 0
5 5

2. We see that Δy becomes smaller when Δx becomes smaller.
3. Hence, lim y 0 for a continuous function.
x 0

CONCLUSION
From the above activity, it is verified that for a function to be continuous at any given point x ,
0
Δy = | f (x + Δ x) – f ( x )| is arbitrarily small provided Δ x is sufficiently small.
0 0

APPLICATION
This activity is helpful in explaining the concept of derivative (left hand or right hand) at any point on the
curve corresponding to a function.

Knowledge Booster
Reasons of Discontinuity:
The discontinuity of a function may be due to the following reasons (It is assumed the function f (x) is defined at x = c)
1. The left hand limit or right hand limit or both may not exist.
2. The left hand limit or right hand limit exist but are not equal.
3. The left hand limit or right hand limit exist and are equal but not equal to f (c).

41