Page 71 - Revised Maths Wisdom Class - 6

Fractions 69

Step 2: Write the quotient as a whole number i.e., 1.
Step 3: Write the remainder as the numerator of the fractional part and the divisor as the denominator of the
2
fractional part i.e., 1 .
3

Remainder
Improper fraction = Quotient = Mixed fractionn
Divisor

ILLUSTRATIONS

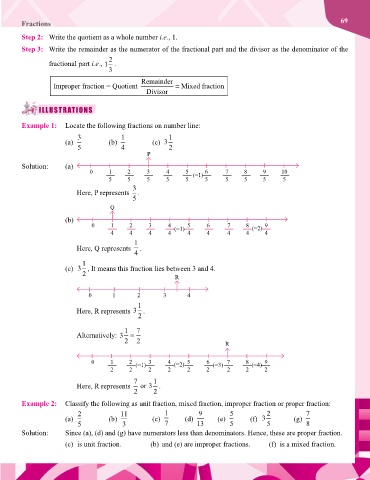
Example 1: Locate the following fractions on number line:
3 1 1
(a) (b) (c) 3
5 4 2
P
Solution: (a)
0 1 2 3 4 5 (=1) 6 7 8 9 10
5 5 5 5 5 5 5 5 5 5
3
Here, P represents .
5
Q
(b)
0 1 2 3 4 (=1) 5 6 7 8 (=2) 9
4 4 4 4 4 4 4 4 4
1
Here, Q represents .
4
1
(c) 3 , It means this fraction lies between 3 and 4.
2 R

0 1 2 3 4
1
Here, R represents 3 .
2

1 7
Alternatively: 3 =
2 2 R

0 1 2 (=1) 3 4 (=2) 5 6 (=3) 7 8 (=4) 9
2 2 2 2 2 2 2 2 2

7 1
Here, R represents or 3 .
2 2
Example 2: Classify the following as unit fraction, mixed fraction, improper fraction or proper fraction:
2 11 1 9 5 2 7
(a) (b) (c) (d) (e) (f) 3 (g)
5 3 7 13 5 5 8
Solution: Since (a), (d) and (g) have numerators less than denominators. Hence, these are proper fraction.

(c) is unit fraction. (b) and (e) are improper fractions. (f) is a mixed fraction.