Page 74 - Revised Maths Wisdom Class - 6

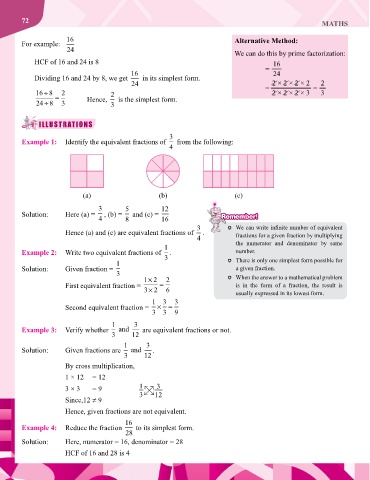
72 MATHS

16 Alternative Method:
For example:
24 We can do this by prime factorization:

HCF of 16 and 24 is 8 16
16 = 24
Dividing 16 and 24 by 8, we get in its simplest form.
24 = 2 × 2 × 2 × 2 = 2
16 8÷ 2 2 2 × 2 × 2 × 3 3
24 8÷ = 3 Hence, 3 is the simplest form.

ILLUSTRATIONS

3
Example 1: Identify the equivalent fractions of from the following:
4

(a) (b) (c)

3 5 12
Solution: Here (a) = , (b) = and (c) =
Rememb
4 8 16 Remember!er!
3 › We can write infinite number of equivalent
Hence (a) and (c) are equivalent fractions of .
4 fractions for a given fraction by multiplying
1 the numerator and denominator by same
Example 2: Write two equivalent fractions of . number.
3
1 › There is only one simplest form possible for
Solution: Given fraction = a given fraction.
3
12× 2 › When the answer to a mathematical problem
First equivalent fraction = = is in the form of a fraction, the result is
32× 6 usually expressed in its lowest form.
1 3 3
Second equivalent fraction = ×=
3 3 9

1 3
Example 3: Verify whether and are equivalent fractions or not.
3 12
1 3
Solution: Given fractions are and .
3 12
By cross multiplication,

1 × 12 = 12
3 × 3 = 9 1 3

Since,12 ≠ 9 3 12
Hence, given fractions are not equivalent.
16
Example 4: Reduce the fraction to its simplest form.
28
Solution: Here, numerator = 16, denominator = 28
HCF of 16 and 28 is 4