Page 73 - Revised Maths Wisdom Class - 6

Fractions 71

4. Write three improper fractions with denominator 5.

5. Convert the following into improper fraction:
1 3 8 14 9
(a) 2 (b) 16 (c) 8 (d) 13 (e) 11
7 5 9 15 100
6. Express the following as mixed fractions:

47 33 89 117 889
(a) (b) (c) (d) (e)
4 2 13 20 17
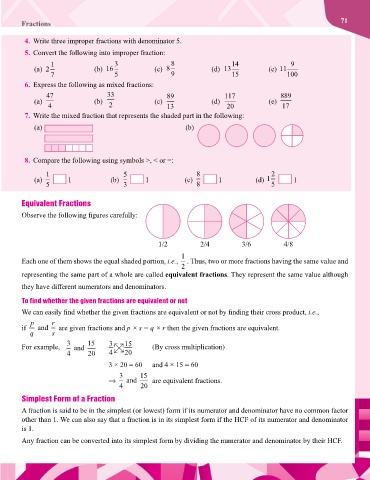
7. Write the mixed fraction that represents the shaded part in the following:
(a) (b)

8. Compare the following using symbols >, < or =:

1 5 8 2
(a) 1 (b) 1 (c) 1 (d) 1 1
5 3 8 5

Equivalent Fractions
Observe the following figures carefully:

1/2 2/4 3/6 4/8

1
Each one of them shows the equal shaded portion, i.e., . Thus, two or more fractions having the same value and
2
representing the same part of a whole are called equivalent fractions. They represent the same value although
they have different numerators and denominators.

To find whether the given fractions are equivalent or not
We can easily find whether the given fractions are equivalent or not by finding their cross product, i.e.,

p r
if and are given fractions and p × s = q × r then the given fractions are equivalent.
q s
For example, 3 and 15 3 15 (By cross multiplication)
4 20 4 20
3 × 20 = 60 and 4 × 15 = 60
3 15
⇒ and are equivalent fractions.
4 20

Simplest Form of a Fraction

A fraction is said to be in the simplest (or lowest) form if its numerator and denominator have no common factor
other than 1. We can also say that a fraction is in its simplest form if the HCF of its numerator and denominator
is 1.

Any fraction can be converted into its simplest form by dividing the numerator and denominator by their HCF.