Page 176 - Maths Skills - 7

174 Maths

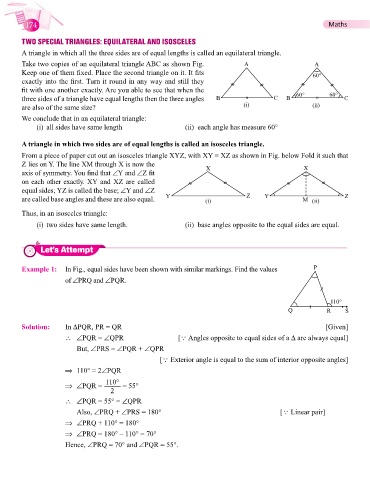
TWO SPECIAL TRIANGLES: EQUILATERAL AND ISOSCELES
A triangle in which all the three sides are of equal lengths is called an equilateral triangle.
Take two copies of an equilateral triangle ABC as shown Fig. A A
Keep one of them fixed. Place the second triangle on it. It fits 60°
exactly into the first. Turn it round in any way and still they
fit with one another exactly. Are you able to see that when the
three sides of a triangle have equal lengths then the three angles B C B 60° 60° C
are also of the same size? (i) (ii)
We conclude that in an equilateral triangle:
(i) all sides have same length (ii) each angle has measure 60°

A triangle in which two sides are of equal lengths is called an isosceles triangle.
From a piece of paper cut out an isosceles triangle XYZ, with XY = XZ as shown in Fig. below Fold it such that
Z lies on Y. The line XM through X is now the
axis of symmetry. You find that ∠Y and ∠Z fit
on each other exactly. XY and XZ are called
equal sides; YZ is called the base; ∠Y and ∠Z
are called base angles and these are also equal. (i) M (ii)

Thus, in an isosceles triangle:
(i) two sides have same length. (ii) base angles opposite to the equal sides are equal.

Let’s Attempt

Example 1: In Fig., equal sides have been shown with similar markings. Find the values

of ∠PRQ and ∠PQR.

Solution: In DPQR, PR = QR [Given]

\ ∠PQR = ∠QPR [Q Angles opposite to equal sides of a D are always equal]
But, ∠PRS = ∠PQR + ∠QPR
[Q Exterior angle is equal to the sum of interior opposite angles]
⇒ 110° = 2∠PQR

⇒ ∠PQR = 110° = 55°
2
\ ∠PQR = 55° = ∠QPR
Also, ∠PRQ + ∠PRS = 180° [Q Linear pair]
⇒ ∠PRQ + 110° = 180°
⇒ ∠PRQ = 180° – 110° = 70°

Hence, ∠PRQ = 70° and ∠PQR = 55°.