Page 180 - Maths Skills - 7

178 Maths

Now, in right DBED in figure, by Pythagoras’ theorem, we have

BD = BE + ED = 15 + 36 = 225 + 1296 = 1521
2
2
2
2
2
∴ BD = 1521 39=

So, BD = 39 m
Hence, the distance between the tops of the buildings is 39 m.
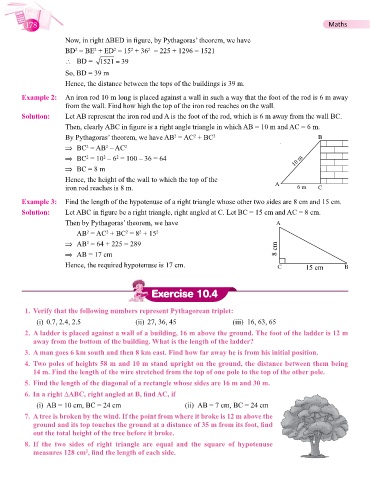
Example 2: An iron rod 10 m long is placed against a wall in such a way that the foot of the rod is 6 m away
from the wall. Find how high the top of the iron rod reaches on the wall.
Solution: Let AB represent the iron rod and A is the foot of the rod, which is 6 m away from the wall BC.
Then, clearly ABC in figure is a right angle triangle in which AB = 10 m and AC = 6 m.
By Pythagoras’ theorem, we have AB = AC + BC 2 B
2
2
⇒ BC = AB – AC 2
2
2
⇒ BC = 10 – 6 = 100 – 36 = 64 10 m
2
2
2
⇒ BC = 8 m
Hence, the height of the wall to which the top of the
iron rod reaches is 8 m. A 6 m C
Example 3: Find the length of the hypotenuse of a right triangle whose other two sides are 8 cm and 15 cm.
Solution: Let ABC in figure be a right triangle, right angled at C. Let BC = 15 cm and AC = 8 cm.
Then by Pythagoras’ theorem, we have A
AB = AC + BC = 8 + 15 2
2
2
2
2
⇒ AB = 64 + 225 = 289
2
⇒ AB = 17 cm 8 cm
Hence, the required hypotenuse is 17 cm. C 15 cm B

Exercise 10.4

1. Verify that the following numbers represent Pythagorean triplet:
(i) 0.7, 2.4, 2.5 (ii) 27, 36, 45 (iii) 16, 63, 65

2. A ladder is placed against a wall of a building, 16 m above the ground. The foot of the ladder is 12 m
away from the bottom of the building. What is the length of the ladder?

3. A man goes 6 km south and then 8 km east. Find how far away he is from his initial position.
4. Two poles of heights 58 m and 10 m stand upright on the ground, the distance between them being
14 m. Find the length of the wire stretched from the top of one pole to the top of the other pole.
5. Find the length of the diagonal of a rectangle whose sides are 16 m and 30 m.
6. In a right DABC, right angled at B, find AC, if
(i) AB = 10 cm, BC = 24 cm (ii) AB = 7 cm, BC = 24 cm

7. A tree is broken by the wind. If the point from where it broke is 12 m above the
ground and its top touches the ground at a distance of 35 m from its foot, find
out the total height of the tree before it broke.
8. If the two sides of right triangle are equal and the square of hypotenuse
measures 128 cm , find the length of each side.
2