Page 177 - Maths Skills - 7

The Triangle and Its Properties 175

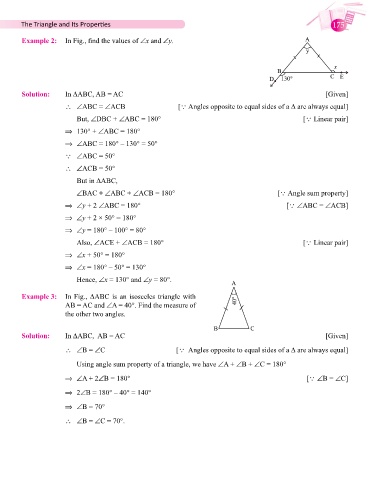
Example 2: In Fig., find the values of ∠x and ∠y.

Solution: In DABC, AB = AC [Given]

\ ∠ABC = ∠ACB [Q Angles opposite to equal sides of a D are always equal]

But, ∠DBC + ∠ABC = 180° [Q Linear pair]
⇒ 130° + ∠ABC = 180°

⇒ ∠ABC = 180° – 130° = 50°
Q ∠ABC = 50°

\ ∠ACB = 50°
But in DABC,

∠BAC + ∠ABC + ∠ACB = 180° [Q Angle sum property]
⇒ ∠y + 2 ∠ABC = 180° [Q ∠ABC = ∠ACB]

⇒ ∠y + 2 × 50° = 180°
⇒ ∠y = 180° – 100° = 80°

Also, ∠ACE + ∠ACB = 180° [Q Linear pair]

⇒ ∠x + 50° = 180°
⇒ ∠x = 180° – 50° = 130°

Hence, ∠x = 130° and ∠y = 80°.

Example 3: In Fig., DABC is an isosceles triangle with
AB = AC and ∠A = 40°. Find the measure of
the other two angles.

Solution: In DABC, AB = AC [Given]

\ ∠B = ∠C [Q Angles opposite to equal sides of a D are always equal]

Using angle sum property of a triangle, we have ∠A + ∠B + ∠C = 180°

⇒ ∠A + 2∠B = 180° [Q ∠B = ∠C]

⇒ 2∠B = 180° – 40° = 140°

⇒ ∠B = 70°

\ ∠B = ∠C = 70°.