Page 174 - Maths Skills - 7

172 Maths

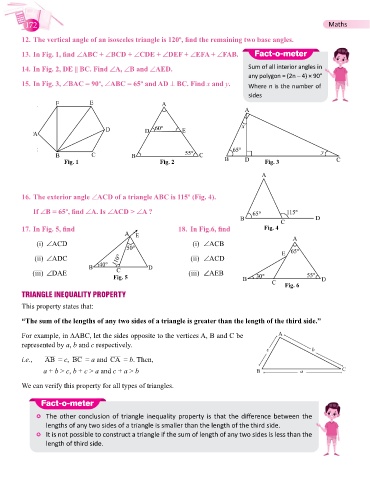
12. The vertical angle of an isosceles triangle is 120º, find the remaining two base angles.

13. In Fig. 1, find ∠ABC + ∠BCD + ∠CDE + ∠DEF + ∠EFA + ∠FAB. Fact-o-meter

14. In Fig. 2, DE || BC. Find ∠A, ∠B and ∠AED. Sum of all interior angles in
any polygon = (2n – 4) × 90°
15. In Fig. 3, ∠BAC = 90º, ∠ABC = 65º and AD ^ BC. Find x and y. Where n is the number of

sides

Fig. 1 Fig. 2 Fig. 3

16. The exterior angle ∠ACD of a triangle ABC is 115º (Fig. 4).

If ∠B = 65º, find ∠A. Is ∠ACD > ∠A ?

17. In Fig. 5, find 18. In Fig.6, find Fig. 4

(i) ∠ACD (i) ∠ACB

(ii) ∠ADC (ii) ∠ACD

(iii) ∠DAE (iii) ∠AEB
Fig. 5
Fig. 6
TRIANGLE INEQUALITY PROPERTY
This property states that:

“The sum of the lengths of any two sides of a triangle is greater than the length of the third side.”

For example, in ∆ABC, let the sides opposite to the vertices A, B and C be A
represented by a, b and c respectively.
c b
i.e., AB = c, BC = a and CA = b. Then,

a + b > c, b + c > a and c + a > b B a C
We can verify this property for all types of triangles.

Fact-o-meter

� The other conclusion of triangle inequality property is that the difference between the
lengths of any two sides of a triangle is smaller than the length of the third side.
� It is not possible to construct a triangle if the sum of length of any two sides is less than the
length of third side.