Page 172 - Maths Skills - 7

170 Maths

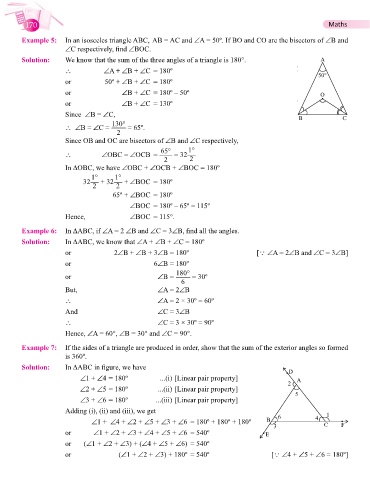
Example 5: In an isosceles triangle ABC, AB = AC and ∠A = 50º. If BO and CO are the bisectors of ∠B and
∠C respectively, find ∠BOC.
Solution: We know that the sum of the three angles of a triangle is 180°. A

\ ∠A + ∠B + ∠C = 180º 50°
or 50º + ∠B + ∠C = 180º
or ∠B + ∠C = 180º – 50º O
or ∠B + ∠C = 130º

Since ∠B = ∠C, B C
\ ∠B = ∠C = 130° = 65º.
2
Since OB and OC are bisectors of ∠B and ∠C respectively,

\ ∠OBC = ∠OCB = 65° = 32 1°
2 2
In DOBC, we have ∠OBC + ∠OCB + ∠BOC = 180º
1° 1°
32 + 32 + ∠BOC = 180º
2 2
65º + ∠BOC = 180º
∠BOC = 180º – 65º = 115º

Hence, ∠BOC = 115°.

Example 6: In DABC, if ∠A = 2 ∠B and ∠C = 3∠B, find all the angles.
Solution: In DABC, we know that ∠A + ∠B + ∠C = 180º
or 2∠B + ∠B + 3∠B = 180º [Q ∠A = 2∠B and ∠C = 3∠B]
or 6∠B = 180º

or ∠B = 180° = 30º
6
But, ∠A = 2∠B
\ ∠A = 2 × 30º = 60º
And ∠C = 3∠B

\ ∠C = 3 × 30º = 90º
Hence, ∠A = 60°, ∠B = 30° and ∠C = 90°.

Example 7: If the sides of a triangle are produced in order, show that the sum of the exterior angles so formed
is 360º.

Solution: In DABC in figure, we have D
∠1 + ∠4 = 180º ...(i) [Linear pair property] 2 A
∠2 + ∠5 = 180º ...(ii) [Linear pair property] 5

∠3 + ∠6 = 180º ...(iii) [Linear pair property]
Adding (i), (ii) and (iii), we get 1
∠1 + ∠4 + ∠2 + ∠5 + ∠3 + ∠6 = 180º + 180º + 180º B 3 6 4 C F
or ∠1 + ∠2 + ∠3 + ∠4 + ∠5 + ∠6 = 540º E
or (∠1 + ∠2 + ∠3) + (∠4 + ∠5 + ∠6) = 540º

or (∠1 + ∠2 + ∠3) + 180º = 540º [Q ∠4 + ∠5 + ∠6 = 180º]