Page 170 - Maths Skills - 7

168 Maths

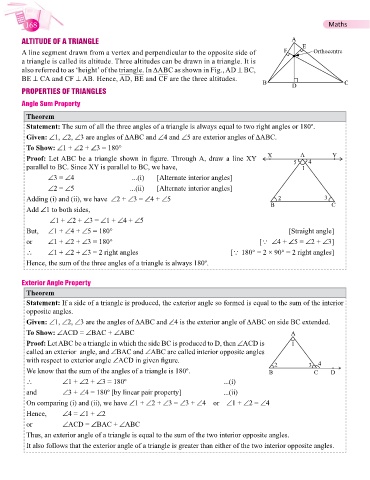
ALTITUDE OF A TRIANGLE
A line segment drawn from a vertex and perpendicular to the opposite side of Orthocentre
a triangle is called its altitude. Three altitudes can be drawn in a triangle. It is
also referred to as ‘height’ of the triangle. In DABC as shown in Fig., AD ^ BC,
BE ^ CA and CF ^ AB. Hence, AD, BE and CF are the three altitudes.

PROPERTIES OF TRIANGLES
Angle Sum Property

Theorem
Statement: The sum of all the three angles of a triangle is always equal to two right angles or 180º.
Given: ∠1, ∠2, ∠3 are angles of DABC and ∠4 and ∠5 are exterior angles of DABC.

To Show: ∠1 + ∠2 + ∠3 = 180°
Proof: Let ABC be a triangle shown in figure. Through A, draw a line XY X 5 A 4 Y
parallel to BC. Since XY is parallel to BC, we have, 1

∠3 = ∠4 ...(i) [Alternate interior angles]
∠2 = ∠5 ...(ii) [Alternate interior angles]
Adding (i) and (ii), we have ∠2 + ∠3 = ∠4 + ∠5 2 3
Add ∠1 to both sides, B C
∠1 + ∠2 + ∠3 = ∠1 + ∠4 + ∠5

But, ∠1 + ∠4 + ∠5 = 180° [Straight angle]
or ∠1 + ∠2 + ∠3 = 180° [Q ∠4 + ∠5 = ∠2 + ∠3]
\ ∠1 + ∠2 + ∠3 = 2 right angles [Q 180° = 2 × 90° = 2 right angles]
Hence, the sum of the three angles of a triangle is always 180º.

Exterior Angle Property
Theorem
Statement: If a side of a triangle is produced, the exterior angle so formed is equal to the sum of the interior
opposite angles.
Given: ∠1, ∠2, ∠3 are the angles of DABC and ∠4 is the exterior angle of DABC on side BC extended.

To Show: ∠ACD = ∠BAC + ∠ABC A
Proof: Let ABC be a triangle in which the side BC is produced to D, then ∠ACD is 1
called an exterior angle, and ∠BAC and ∠ABC are called interior opposite angles
with respect to exterior angle ∠ACD in given figure. 2 3 4

We know that the sum of the angles of a triangle is 180º. B C D
\ ∠1 + ∠2 + ∠3 = 180º ...(i)
and ∠3 + ∠4 = 180º [by linear pair property] ...(ii)
On comparing (i) and (ii), we have ∠1 + ∠2 + ∠3 = ∠3 + ∠4 or ∠1 + ∠2 = ∠4
Hence, ∠4 = ∠1 + ∠2
or ∠ACD = ∠BAC + ∠ABC

Thus, an exterior angle of a triangle is equal to the sum of the two interior opposite angles.
It also follows that the exterior angle of a triangle is greater than either of the two interior opposite angles.