Page 95 - Mathematics Class - IX

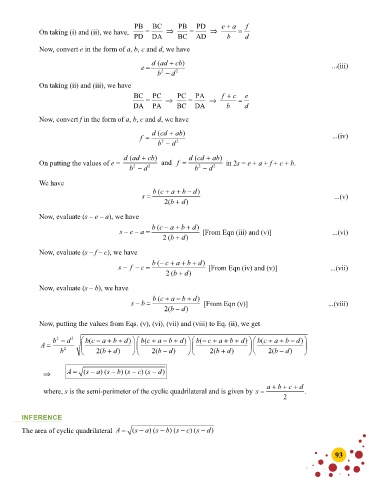
PB BC PB PD e + a f
On taking (i) and (ii), we have, = ⇒ = ⇒ =
PD DA BC AD b d
Now, convert e in the form of a, b, c and d, we have

dad +( cb)
e = 2 2 ...(iii)
b − d
On taking (ii) and (iii), we have
BC = PC ⇒ PC = PA ⇒ f + c = e
DA PA BC DA b d

Now, convert f in the form of a, b, c and d, we have
dcd +( ab)
f = 2 2 ...(iv)
b − d

dad( + cb) dcd( + ab)
On putting the values of e = 2 2 and f = 2 2 in 2s = e + a + f + c + b.
b − d b − d

We have
bc ++ −( a b d)
s = ...(v)
d
( 2 b + )

Now, evaluate (s – e – a), we have
bc −+ +( a b d)
a
s −− = [From Eqn (iii) and (v)] ...(vi)
e
d
2 ( b + )
Now, evaluate (s – f – c), we have
b −+ ++( c a b d)
s − f − = [From Eqn (iv) and (v)] ...(vii)
c
d
2 ( b + )
Now, evaluate (s – b), we have
bc +− +( ab d)
b
s −= [From Eqn (v)] ...(viii)
d
( 2 b − )
Now, putting the values from Eqs. (v), (vi), (vii) and (viii) to Eq. (ii), we get

b
bc +− + ) 
c
b
d 
a
2
d   (
(
bc − ++ )
b − d 2  ( a b d   ( ab d  b −+ aa ++ ) bc ++ − )
A =        
d
b 2  2( b + )   2( b − )   ( 2 b + )   ( 2 b − ) 
d
d
d
⇒ A = ( s − as −) ( bs −) ( cs −) ( d)
c
b
a ++ + d
where, s is the semi-perimeter of the cyclic quadrilateral and is given by s = .
2
INFERENCE
The area of cyclic quadrilateral A = ( s − as −) ( bs −) ( cs −) ( d)
93