Page 93 - Mathematics Class - IX

PROJECT 3

AIM

To develop Heron’s formula for area of a triangle.
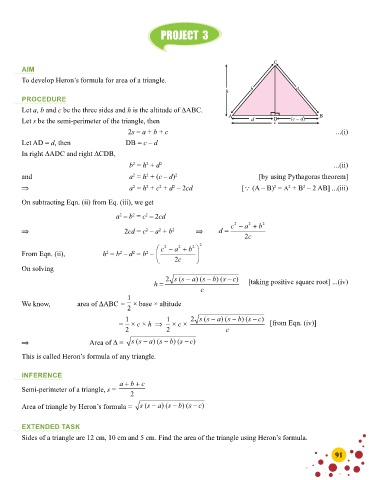
b a
h
PROCEDURE
Let a, b and c be the three sides and h is the altitude of ∆ABC.
Let s be the semi-perimeter of the triangle, then A d D (c– d ) B
2s = a + b + c ...(i)
Let AD = d, then DB = c – d

In right ∆ADC and right ∆CDB,
b = h + d 2 ...(ii)
2
2
and a = h + (c – d) [by using Pythagoras theorem]
2
2
2
⇒ a = h + c + d – 2cd [ (A – B) = A + B – 2 AB] ...(iii)
2
2
2
2
2
2
2
On subtracting Eqn. (ii) from Eq. (iii), we get
a – b = c – 2cd
2
2
2
2
2
c − a + b 2
⇒ 2cd = c – a + b ⇒ d =
2
2
2
c 2
2
2
2
2
From Eqn. (ii), h = b – d = b –  c − a + b  2
2
2
2


 c 2 
On solving
2 ss −( as −)( bs c−)( )
h = [taking positive square root] ...(iv)
c
1
We know, area of ∆ABC = × base × altitude
2
1 1 2 ss( − as) ( − bs c) ( − )
= × c × h ⇒ × c × [from Eqn. (iv)]
2 2 c
⇒ Area of ∆ = ss( − as) ( − bs c) ( − )
This is called Heron’s formula of any triangle.
INFERENCE
b
a ++ c
Semi-perimeter of a triangle, s =
2
Area of triangle by Heron’s formula = ss( − as) ( − bs c) ( − )
EXTENDED TASK
Sides of a triangle are 12 cm, 10 cm and 5 cm. Find the area of the triangle using Heron’s formula.
91