Page 94 - Mathematics Class - IX

PROJECT 4

AIM

Development of formula for the area of a cyclic quadrilateral.

PROCEDURE
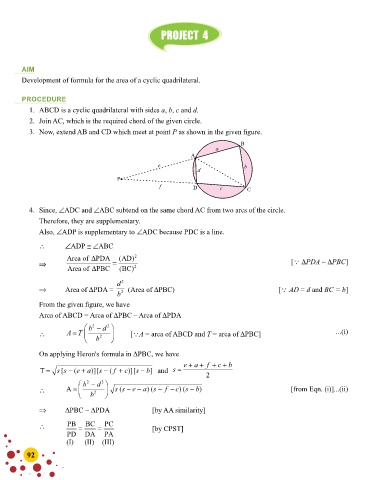
1. ABCD is a cyclic quadrilateral with sides a, b, c and d.
2. Join AC, which is the required chord of the given circle.
3. Now, extend AB and CD which meet at point P as shown in the given figure.

B
a
A
e b
d
P
f D c C

4. Since, ∠ADC and ∠ABC subtend on the same chord AC from two arcs of the circle.
Therefore, they are supplementary.

Also, ∠ADP is supplementary to ∠ADC because PDC is a line.

\ ∠ADP ≅ ∠ABC
Area of PDA∆ ( AD) 2
⇒ = 2 [ ∆PDA ∼ ∆PBC]
Area of PBC∆ ( BC)

d 2
⇒ Area of ∆PDA = 2 (Area of ∆PBC) [ AD = d and BC = b]
b
From the given figure, we have
Area of ABCD = Area of ∆PBC – Area of ∆PDA

2
2
 b − d 
=
\ AT  2  [A = area of ABCD and T = area of ∆PBC] ...(i)
 b 
On applying Heron's formula in ∆PBC, we have
c
a
e ++ f ++ b
T = ss[ − e( + a)][ s − f( + c)][ s − b] and s = 2

 b 2 − d 2 
e
)
)
\ A =  b 2   ss ( −− a s ( − f − cs ( − b) [from Eqn. (i)]...(ii)


⇒ ∆PBC ∼ ∆PDA [by AA similarity]
\ PB = BC = PC [by CPST]
PD DA PA
(I) (II) (III)

92