Page 99 - Mathematics Class - IX

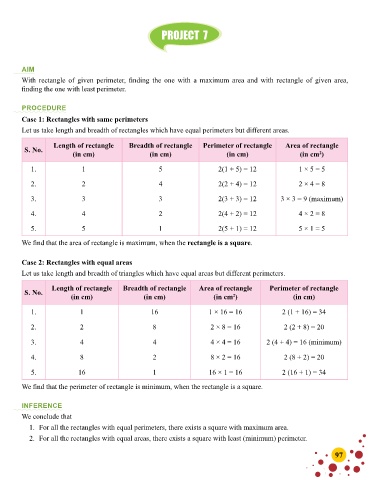
PROJECT 7

AIM

With rectangle of given perimeter, finding the one with a maximum area and with rectangle of given area,
finding the one with least perimeter.

PROCEDURE

Case 1: Rectangles with same perimeters
Let us take length and breadth of rectangles which have equal perimeters but different areas.

Length of rectangle Breadth of rectangle Perimeter of rectangle Area of rectangle
S. No.
(in cm) (in cm) (in cm) (in cm )
2
1. 1 5 2(1 + 5) = 12 1 × 5 = 5

2. 2 4 2(2 + 4) = 12 2 × 4 = 8

3. 3 3 2(3 + 3) = 12 3 × 3 = 9 (maximum)

4. 4 2 2(4 + 2) = 12 4 × 2 = 8

5. 5 1 2(5 + 1) = 12 5 × 1 = 5

We find that the area of rectangle is maximum, when the rectangle is a square.

Case 2: Rectangles with equal areas
Let us take length and breadth of triangles which have equal areas but different perimeters.

Length of rectangle Breadth of rectangle Area of rectangle Perimeter of rectangle
S. No.
(in cm) (in cm) (in cm ) (in cm)
2
1. 1 16 1 × 16 = 16 2 (1 + 16) = 34

2. 2 8 2 × 8 = 16 2 (2 + 8) = 20

3. 4 4 4 × 4 = 16 2 (4 + 4) = 16 (minimum)

4. 8 2 8 × 2 = 16 2 (8 + 2) = 20

5. 16 1 16 × 1 = 16 2 (16 + 1) = 34

We find that the perimeter of rectangle is minimum, when the rectangle is a square.

INFERENCE
We conclude that
1. For all the rectangles with equal perimeters, there exists a square with maximum area.
2. For all the rectangles with equal areas, there exists a square with least (minimum) perimeter.

97