Page 127 - Revised Maths Wisdom Class - 6

Playing With Construction 125

Squares and Rectangles
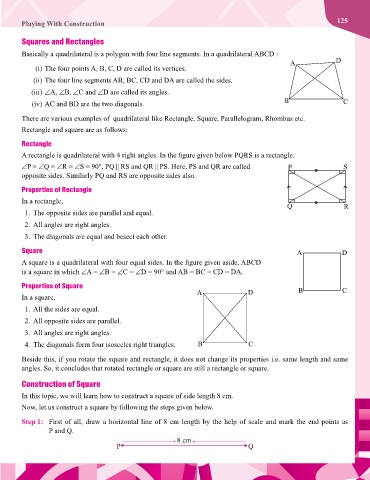
Basically a quadrilateral is a polygon with four line segments. In a quadrilateral ABCD :
D
A
(i) The four points A, B, C, D are called its vertices.
(ii) The four line segments AB, BC, CD and DA are called the sides.
(iii) ∠A, ∠B, ∠C and ∠D are called its angles.
(iv) AC and BD are the two diagonals. B C

There are various examples of quadrilateral like Rectangle, Square, Parallelogram, Rhombus etc.

Rectangle and square are as follows:

Rectangle
A rectangle is quadrilateral with 4 right angles. In the figure given below PQRS is a rectangle.
∠P = ∠Q = ∠R = ∠S = 90°, PQ || RS and QR || PS. Here, PS and QR are called P S
opposite sides. Similarly PQ and RS are opposite sides also.

Properties of Rectangle
In a rectangle,
Q R
1. The opposite sides are parallel and equal.
2. All angles are right angles.

3. The diagonals are equal and besect each other.

Square A D
A square is a quadrilateral with four equal sides. In the figure given aside, ABCD
is a square in which ∠A = ∠B = ∠C = ∠D = 90° and AB = BC = CD = DA.

Properties of Square B C
In a square, A D

1. All the sides are equal.
2. All opposite sides are parallel.

3. All angles are right angles.
4. The diagonals form four isosceles right triangles. B C

Beside this, if you rotate the square and rectangle, it does not change its properties i.e. same length and same
angles. So, it concludes that rotated rectangle or square are still a rectangle or square.

Construction of Square

In this topic, we will learn how to construct a square of side length 8 cm.
Now, let us construct a square by following the steps given below.

Step 1: First of all, draw a horizontal line of 8 cm length by the help of scale and mark the end points as
P and Q.
8 cm
P Q