Page 89 - Maths Skills - 8

Factorisation 87

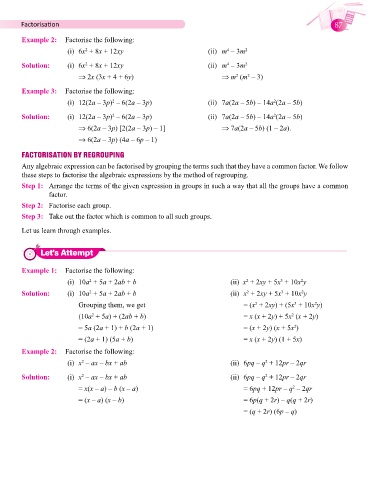
Example 2: Factorise the following:

(i) 6x + 8x + 12xy (ii) m – 3m 2
4
2
Solution: (i) 6x + 8x + 12xy (ii) m – 3m 2
4
2
⇒ 2x (3x + 4 + 6y) ⇒ m (m – 3)
2
2
Example 3: Factorise the following:
(i) 12(2a – 3p) – 6(2a – 3p) (ii) 7a(2a – 5b) – 14a (2a – 5b)
2
2
Solution: (i) 12(2a – 3p) – 6(2a – 3p) (ii) 7a(2a – 5b) – 14a (2a – 5b)
2
2
⇒ 6(2a – 3p) [2(2a – 3p) – 1] ⇒ 7a(2a – 5b) (1 – 2a).
⇒ 6(2a – 3p) (4a – 6p – 1)

FACTORISATION BY REGROUPING
Any algebraic expression can be factorised by grouping the terms such that they have a common factor. We follow
these steps to factorise the algebraic expressions by the method of regrouping.
Step 1: Arrange the terms of the given expression in groups in such a way that all the groups have a common
factor.
Step 2: Factorise each group.

Step 3: Take out the factor which is common to all such groups.
Let us learn through examples.

Let’s Attempt

Example 1: Factorise the following:
(i) 10a + 5a + 2ab + b (ii) x + 2xy + 5x + 10x y
3
2
2
2
Solution: (i) 10a + 5a + 2ab + b (ii) x + 2xy + 5x + 10x y
2
3
2
2
Grouping them, we get = (x + 2xy) + (5x + 10x y)
2
3
2
(10a + 5a) + (2ab + b) = x (x + 2y) + 5x (x + 2y)
2
2
= 5a (2a + 1) + b (2a + 1) = (x + 2y) (x + 5x )
2
= (2a + 1) (5a + b) = x (x + 2y) (1 + 5x)
Example 2: Factorise the following:
(i) x – ax – bx + ab (ii) 6pq – q + 12pr – 2qr
2
2
Solution: (i) x – ax – bx + ab (ii) 6pq – q + 12pr – 2qr
2
2
= x(x – a) – b (x – a) = 6pq + 12pr – q – 2qr
2
= (x – a) (x – b) = 6p(q + 2r) – q(q + 2r)
= (q + 2r) (6p – q)