Page 94 - Maths Skills - 8

92 Maths

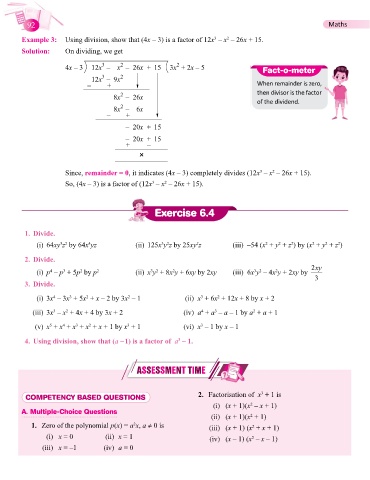
Example 3: Using division, show that (4x – 3) is a factor of 12x – x – 26x + 15.
2
3
Solution: On dividing, we get

3
2
4– 3x 12x – x –26x +15 3x 2 + 2– 5x Fact-o-meter
3 2
12x –9x When remainder is zero,
– +
then divisor is the factor
2
8x – 26x
of the dividend.
2
8x – 6x
– +
– 20x +15
– 20x +15
+ –
×

Since, remainder = 0, it indicates (4x – 3) completely divides (12x – x – 26x + 15).
2
3
So, (4x – 3) is a factor of (12x – x – 26x + 15).
2
3

Exercise 6.4

1. Divide.
(i) 64xy z by 64x yz (ii) 125x y z by 25xy z (iii) –54 (x + y + z ) by (x + y + z )
2
3 2
2
2
2
2
2
2
3 2
4
2. Divide.
2xy
(i) p – p + 5p by p 2 (ii) x y + 8x y + 6xy by 2xy (iii) 6x y – 4x y + 2xy by
2
3 2
3 2
4
2
2
3
3
3. Divide.
(i) 3x – 3x + 5x + x – 2 by 3x – 1 (ii) x + 6x + 12x + 8 by x + 2
2
3
2
3
2
4
(iii) 3x – x + 4x + 4 by 3x + 2 (iv) a + a – a – 1 by a + a + 1
3
4
3
2
2
(v) x + x + x + x + x + 1 by x + 1 (vi) x – 1 by x – 1
3
4
5
2
3
3
4. Using division, show that (a – 1) is a factor of a – 1.
3
ASSESSMENT TIME
2. Factorisation of x + 1 is
3
COMPETENCY BASED QUESTIONS
(i) (x + 1)(x – x + 1)
2
A. Multiple-Choice Questions
(ii) (x + 1)(x + 1)
2
1. Zero of the polynomial p(x) = a x, a ≠ 0 is (iii) (x + 1) (x + x + 1)
2
2
(i) x = 0 (ii) x = 1 (iv) (x – 1) (x – x – 1)
2
(iii) x = –1 (iv) a = 0