Page 93 - Maths Skills - 8

Factorisation 91

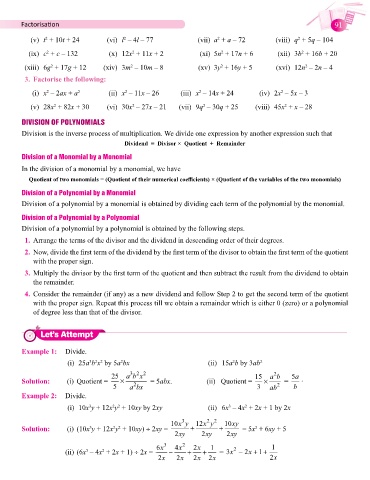
(v) t + 10t + 24 (vi) l – 4l – 77 (vii) a + a – 72 (viii) q + 5q – 104
2
2
2
2
(ix) c + c – 132 (x) 12x + 11x + 2 (xi) 5n + 17n + 6 (xii) 3b + 16b + 20
2
2
2
2
(xiii) 6g + 17g + 12 (xiv) 3m – 10m – 8 (xv) 3y + 16y + 5 (xvi) 12n – 2n – 4
2
2
2
2
3. Factorise the following:
(i) x – 2ax + a 2 (ii) x – 11x – 26 (iii) x – 14x + 24 (iv) 2x – 5x – 3
2
2
2
2
(v) 28x + 82x + 30 (vi) 30x – 27x – 21 (vii) 9q – 30q + 25 (viii) 45x + x – 28
2
2
2
2
DIVISION OF POLYNOMIALS
Division is the inverse process of multiplication. We divide one expression by another expression such that
Dividend = Divisor × Quotient + Remainder
Division of a Monomial by a Monomial
In the division of a monomial by a monomial, we have
Quotient of two monomials = (Quotient of their numerical coefficients) × (Quotient of the variables of the two monomials)
Division of a Polynomial by a Monomial
Division of a polynomial by a monomial is obtained by dividing each term of the polynomial by the monomial.

Division of a Polynomial by a Polynomial
Division of a polynomial by a polynomial is obtained by the following steps.
1. Arrange the terms of the divisor and the dividend in descending order of their degrees.

2. Now, divide the first term of the dividend by the first term of the divisor to obtain the first term of the quotient
with the proper sign.
3. Multiply the divisor by the first term of the quotient and then subtract the result from the dividend to obtain
the remainder.

4. Consider the remainder (if any) as a new dividend and follow Step 2 to get the second term of the quotient
with the proper sign. Repeat this process till we obtain a remainder which is either 0 (zero) or a polynomial
of degree less than that of the divisor.

Let’s Attempt

Example 1: Divide.

(i) 25a b x by 5a bx (ii) 15a b by 3ab 2
2
2
3 2 2
32 2
2
Solution: (i) Quotient = 25 × ab x = 5abx. (ii) Quotient = 15 × ab = 5a ·
2
5 abx 3 ab 2 b
Example 2: Divide.
(i) 10x y + 12x y + 10xy by 2xy (ii) 6x – 4x + 2x + 1 by 2x
2
3
2 2
3
10xy 12xy 10xy
2 2
3
Solution: (i) (10x y + 12x y + 10xy) ÷ 2xy = + + = 5x + 6xy + 5
2
3
2 2
2xy 2xy 2xy
6x 3 4x 2 2x 1 1
(ii) (6x – 4x + 2x + 1) ÷ 2x = − + + = 3x − 2x + +
3
2
2
1
2x 2x 2x 2x 2x