Page 92 - Maths Skills - 8

90 Maths

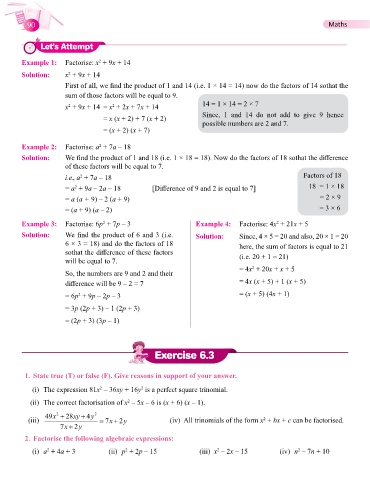
Let’s Attempt

Example 1: Factorise: x + 9x + 14
2
Solution: x + 9x + 14
2
First of all, we find the product of 1 and 14 (i.e. 1 × 14 = 14) now do the factors of 14 sothat the
sum of those factors will be equal to 9.
x + 9x + 14 = x + 2x + 7x + 14 14 = 1 × 14 = 2 × 7
2
2
= x (x + 2) + 7 (x + 2) Since, 1 and 14 do not add to give 9 hence
possible numbers are 2 and 7.
= (x + 2) (x + 7)

Example 2: Factorise: a + 7a – 18
2
Solution: We find the product of 1 and 18 (i.e. 1 × 18 = 18). Now do the factors of 18 sothat the difference
of these factors will be equal to 7.

, a + 7a – 18 Factors of 18
2
= a + 9a – 2a – 18 [Difference of 9 and 2 is equal to 7] 18 = 1 × 18
2
= a (a + 9) – 2 (a + 9) = 2 × 9
= (a + 9) (a – 2) = 3 × 6

Example 3: Factorise: 6p + 7p – 3 Example 4: Factorise: 4x + 21x + 5
2
2
Solution: We find the product of 6 and 3 (i.e. Solution: Since, 4 × 5 = 20 and also, 20 × 1 = 20
6 × 3 = 18) and do the factors of 18 here, the sum of factors is equal to 21
sothat the difference of these factors (i.e. 20 + 1 = 21)
will be equal to 7.
2
So, the numbers are 9 and 2 and their = 4x + 20x + x + 5
difference will be 9 – 2 = 7 = 4x (x + 5) + 1 (x + 5)

= 6p + 9p – 2p – 3 = (x + 5) (4x + 1)
2
= 3p (2p + 3) – 1 (2p + 3)

= (2p + 3) (3p – 1)

Exercise 6.3

1. State true (T) or false (F). Give reasons in support of your answer.

(i) The expression 81x – 36xy + 16y is a perfect square trinomial.
2
2
(ii) The correct factorisation of x – 5x – 6 is (x + 6) (x – 1).
2
2
(iii) 49x 28xy 4y 2 7x 2y (iv) All trinomials of the form x + bx + c can be factorised.
2
7x 2y
2. Factorise the following algebraic expressions:

(i) a + 4a + 3 (ii) p + 2p – 15 (iii) x – 2x – 15 (iv) n – 7n + 10
2
2
2
2