Page 163 - Maths Skills - 8

Understanding Quadrilaterals 161

5. Three angles of a quadrilateral are in the ratio 3 : 2 : 1. The sum of the smallest and the greatest angle
is 180°. Find all the angles of a quadrilateral.
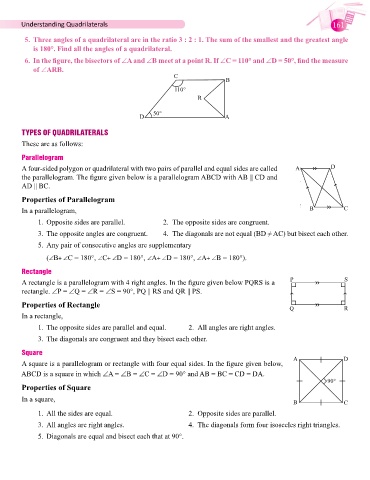
6. In the figure, the bisectors of ∠A and ∠B meet at a point R. If ∠C = 110° and ∠D = 50°, find the measure
of ∠ARB.
C
B
110°
R

D 50° A

TYPES OF QUADRILATERALS

These are as follows:

Parallelogram
A four-sided polygon or quadrilateral with two pairs of parallel and equal sides are called A D
the parallelogram. The figure given below is a parallelogram ABCD with AB || CD and
AD || BC.

Properties of Parallelogram
In a parallelogram, B C

1. Opposite sides are parallel. 2. The opposite sides are congruent.
3. The opposite angles are congruent. 4. The diagonals are not equal (BD ≠ AC) but bisect each other.

5. Any pair of consecutive angles are supplementary
(∠B+ ∠C = 180°, ∠C+ ∠D = 180°, ∠A+ ∠D = 180°, ∠A+ ∠B = 180°).

Rectangle
A rectangle is a parallelogram with 4 right angles. In the figure given below PQRS is a P S
rectangle. ∠P = ∠Q = ∠R = ∠S = 90°, PQ || RS and QR || PS.

Properties of Rectangle Q R
In a rectangle,
1. The opposite sides are parallel and equal. 2. All angles are right angles.

3. The diagonals are congruent and they bisect each other.

Square
A square is a parallelogram or rectangle with four equal sides. In the figure given below, A D
ABCD is a square in which ∠A = ∠B = ∠C = ∠D = 90° and AB = BC = CD = DA.
90°
Properties of Square
In a square, B C

1. All the sides are equal. 2. Opposite sides are parallel.
3. All angles are right angles. 4. The diagonals form four isosceles right triangles.

5. Diagonals are equal and bisect each that at 90°.