Page 162 - Maths Skills - 8

160 Maths

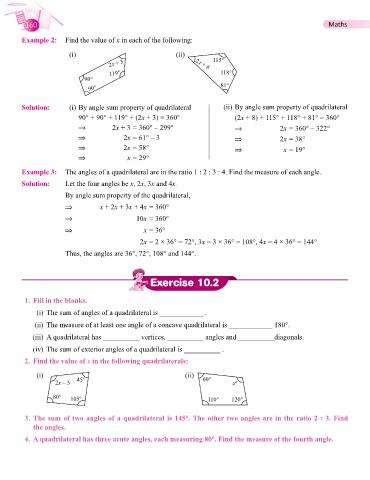
Example 2: Find the value of x in each of the following:

(i) (ii)
2x + 3 2x + 8 115°
119° 118°
90°
90° 81°

Solution: (i) By angle sum property of quadrilateral (ii) By angle sum property of quadrilateral
90° + 90° + 119° + (2x + 3) = 360° (2x + 8) + 115° + 118° + 81° = 360°
⇒ 2x + 3 = 360° – 299° ⇒ 2x = 360° – 322°
⇒ 2x = 61° – 3 ⇒ 2x = 38°

⇒ 2x = 58° ⇒ x = 19°
⇒ x = 29°
Example 3: The angles of a quadrilateral are in the ratio 1 : 2 : 3 : 4. Find the measure of each angle.

Solution: Let the four angles be x, 2x, 3x and 4x.
By angle sum property of the quadrilateral,

⇒ x + 2x + 3x + 4x = 360°
⇒ 10x = 360°
⇒ x = 36°

2x = 2 × 36° = 72°, 3x = 3 × 36° = 108°, 4x = 4 × 36° = 144°
Thus, the angles are 36°, 72°, 108° and 144°.

Exercise 10.2

1. Fill in the blanks.
(i) The sum of angles of a quadrilateral is ____________ .

(ii) The measure of at least one angle of a concave quadrilateral is ____________ 180°.
(iii) A quadrilateral has __________ vertices, __________ angles and __________diagonals.

(iv) The sum of exterior angles of a quadrilateral is __________ .
2. Find the value of x in the following quadrilaterals:

(i) (ii)
2x – 5 45° 60° x°

80° 105° 110° 120°

3. The sum of two angles of a quadrilateral is 145°. The other two angles are in the ratio 2 : 3. Find
the angles.

4. A quadrilateral has three acute angles, each measuring 80°. Find the measure of the fourth angle.