Page 166 - Maths Skills - 8

164 Maths

Let’s Attempt

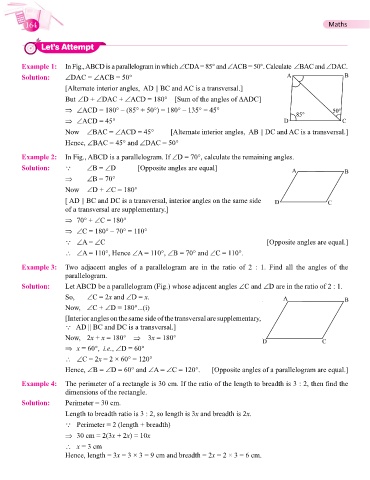
Example 1: In Fig., ABCD is a parallelogram in which ∠CDA = 85° and ∠ACB = 50°. Calculate ∠BAC and ∠DAC.
Solution: ∠DAC = ∠ACB = 50°
[Alternate interior angles, AD || BC and AC is a transversal.]
But ∠D + ∠DAC + ∠ACD = 180° [Sum of the angles of DADC]

⇒ ∠ACD = 180° – (85° + 50°) = 180° – 135° = 45°
⇒ ∠ACD = 45°
Now ∠BAC = ∠ACD = 45° [Alternate interior angles, AB || DC and AC is a transversal.]
Hence, ∠BAC = 45° and ∠DAC = 50°

Example 2: In Fig., ABCD is a parallelogram. If ∠D = 70°, calculate the remaining angles.

Solution:  ∠B = ∠D [Opposite angles are equal]
⇒ ∠B = 70°
Now ∠D + ∠C = 180°
[ AD || BC and DC is a transversal, interior angles on the same side
of a transversal are supplementary.]
⇒ 70° + ∠C = 180°

⇒ ∠C = 180° – 70° = 110°
 ∠A = ∠C [Opposite angles are equal.]
∴ ∠A = 110°, Hence ∠A = 110°, ∠B = 70° and ∠C = 110°.

Example 3: Two adjacent angles of a parallelogram are in the ratio of 2 : 1. Find all the angles of the
parallelogram.
Solution: Let ABCD be a parallelogram (Fig.) whose adjacent angles ∠C and ∠D are in the ratio of 2 : 1.
So, ∠C = 2x and ∠D = x.
Now, ∠C + ∠D = 180°...(i)

[Interior angles on the same side of the transversal are supplementary,
 AD || BC and DC is a transversal.]

Now, 2x + x = 180° ⇒ 3x = 180°
⇒ x = 60°, i.e., ∠D = 60°
∴ ∠C = 2x = 2 × 60° = 120°
Hence, ∠B = ∠D = 60° and ∠A = ∠C = 120°. [Opposite angles of a parallelogram are equal.]

Example 4: The perimeter of a rectangle is 30 cm. If the ratio of the length to breadth is 3 : 2, then find the
dimensions of the rectangle.
Solution: Perimeter = 30 cm.
Length to breadth ratio is 3 : 2, so length is 3x and breadth is 2x.
 Perimeter = 2 (length + breadth)

⇒ 30 cm = 2(3x + 2x) = 10x
∴ x = 3 cm
Hence, length = 3x = 3 × 3 = 9 cm and breadth = 2x = 2 × 3 = 6 cm.