Page 161 - Maths Skills - 8

Understanding Quadrilaterals 159

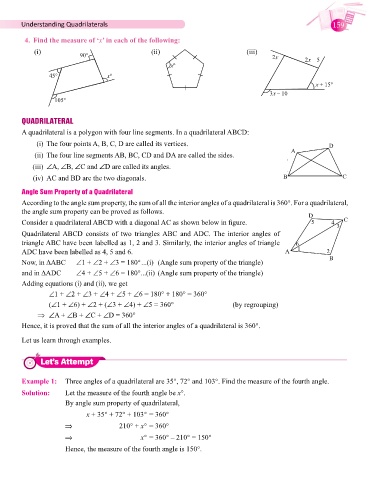
4. Find the measure of ‘x’ in each of the following:

(i) 90° (ii) (iii) 2x

x° 2x – 5
45° x°
x + 15°
3x – 10
105°

QUADRILATERAL
A quadrilateral is a polygon with four line segments. In a quadrilateral ABCD:
(i) The four points A, B, C, D are called its vertices. D

(ii) The four line segments AB, BC, CD and DA are called the sides. A
(iii) ∠A, ∠B, ∠C and ∠D are called its angles.

(iv) AC and BD are the two diagonals. B C

Angle Sum Property of a Quadrilateral
According to the angle sum property, the sum of all the interior angles of a quadrilateral is 360°. For a quadrilateral,
the angle sum property can be proved as follows. D

Consider a quadrilateral ABCD with a diagonal AC as shown below in figure. 5 4 3 C
Quadrilateral ABCD consists of two triangles ABC and ADC. The interior angles of
triangle ABC have been labelled as 1, 2 and 3. Similarly, the interior angles of triangle 6
ADC have been labelled as 4, 5 and 6. A 1 2
Now, in ∆ABC ∠1 + ∠2 + ∠3 = 180° ...(i) (Angle sum property of the triangle) B
and in ∆ADC ∠4 + ∠5 + ∠6 = 180° ...(ii) (Angle sum property of the triangle)
Adding equations (i) and (ii), we get

∠1 + ∠2 + ∠3 + ∠4 + ∠5 + ∠6 = 180° + 180° = 360°
(∠1 + ∠6) + ∠2 + (∠3 + ∠4) + ∠5 = 360° (by regrouping)
⇒ ∠A + ∠B + ∠C + ∠D = 360°
Hence, it is proved that the sum of all the interior angles of a quadrilateral is 360°.

Let us learn through examples.

Let’s Attempt

Example 1: Three angles of a quadrilateral are 35°, 72° and 103°. Find the measure of the fourth angle.
Solution: Let the measure of the fourth angle be x°.
By angle sum property of quadrilateral,
x + 35° + 72° + 103° = 360°

⇒ 210° + x° = 360°
⇒ x° = 360° – 210° = 150°

Hence, the measure of the fourth angle is 150°.