Page 159 - Maths Skills - 8

Understanding Quadrilaterals 157

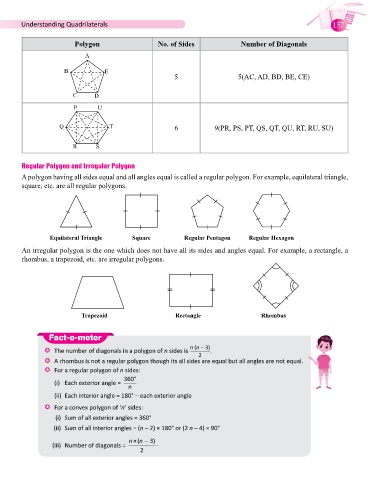
Polygon No. of Sides Number of Diagonals

A

B E
5 5(AC, AD, BD, BE, CE)

C D
P U

Q T 6 9(PR, PS, PT, QS, QT, QU, RT, RU, SU)

R S

Regular Polygon and Irregular Polygon
A polygon having all sides equal and all angles equal is called a regular polygon. For example, equilateral triangle,
square, etc. are all regular polygons.

Equilateral Triangle Square Regular Pentagon Regular Hexagon
An irregular polygon is the one which does not have all its sides and angles equal. For example, a rectangle, a
rhombus, a trapezoid, etc. are irregular polygons.

Trapezoid Rectangle Rhombus

Fact-o-meter
� The number of diagonals in a polygon of n sides is n (n – 3) .
2
� A rhombus is not a regular polygon though its all sides are equal but all angles are not equal.
� For a regular polygon of n sides:
360°
(i) Each exterior angle =
n
(ii) Each interior angle = 180° – each exterior angle
� For a convex polygon of ‘n’ sides:
(i) Sum of all exterior angles = 360°
(ii) Sum of all interior angles = (n – 2) × 180° or (2 n – 4) × 90°

n×( − 3)
n
(iii) Number of diagonals = .
2