Page 145 - Maths Skills - 7

Perimeter and Area 143

AREA OF A CIRCLE
Activity
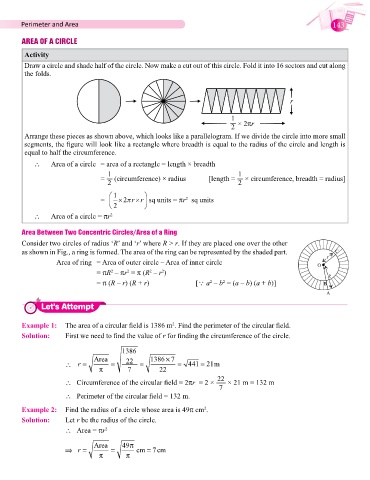
Draw a circle and shade half of the circle. Now make a cut out of this circle. Fold it into 16 sectors and cut along
the folds.

r

1
2 × 2pr
Arrange these pieces as shown above, which looks like a parallelogram. If we divide the circle into more small
segments, the figure will look like a rectangle where breadth is equal to the radius of the circle and length is
equal to half the circumference.

\ Area of a circle = area of a rectangle = length × breadth
1 1
= 2 (circumference) × radius [length = × circumference, breadth = radius]
2
 1 
×
=  × 2πrr sq units = pr sq units
2

 2 
\ Area of a circle = pr 2
Area Between Two Concentric Circles/Area of a Ring
Consider two circles of radius ‘R’ and ‘r’ where R > r. If they are placed one over the other
as shown in Fig., a ring is formed. The area of the ring can be represented by the shaded part.
Area of ring = Area of outer circle – Area of inner circle
= pR – pr = p (R – r )
2
2
2
2
= p (R – r) (R + r) [Q a – b = (a – b) (a + b)]
2
2
Let’s Attempt

Example 1: The area of a circular field is 1386 m . Find the perimeter of the circular field.
2
Solution: First we need to find the value of r for finding the circumference of the circle.

1386
Area 22 1386 × 7
\ r = = = = 441 = 21 m
π 7 22

\ Circumference of the circular field = 2pr = 2 × 22 × 21 m = 132 m
7
\ Perimeter of the circular field = 132 m.

Example 2: Find the radius of a circle whose area is 49p cm .
2
Solution: Let r be the radius of the circle.
\ Area = pr 2

Area 49 π
7
⇒ r = = cm = cm
π π