Page 143 - Maths Skills - 7

Perimeter and Area 141

9. Find the area of the rhombus, whose diagonals are as follows:

(i) d = 15 cm, d = 12 cm (ii) d = 20 cm, d = 22 cm
1 2 1 2
(iii) d = 15 m, d = 10 m (iv) d = 5 cm, d = 300 cm
1 2 1 2
10. One of the diagonals of a rhombus is 24 cm and each side is 20 cm, then find the area of the rhombus.

11. The measure of one of the parallel sides of a trapezium is 4 cm more than the other. If the area of a
trapezium is 80 cm and distance between the parallel sides is 10 cm, find the length of parallel sides.
2
12. The parallel sides of a trapezium are in the ratio 5 : 3 and the other sides are 10 cm each. If the
perimeter of trapezium is 92 cm and distance between its parallel sides is 12 cm, find the length of the
parallel sides and area of the trapezium.

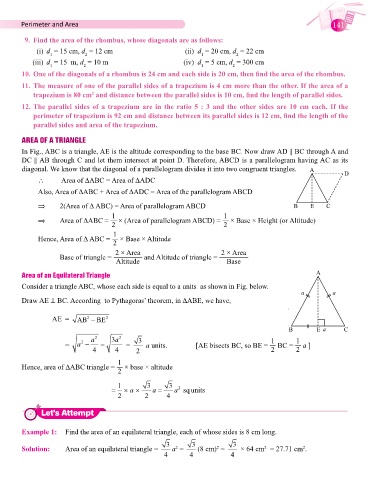
AREA OF A TRIANGLE
In Fig., ABC is a triangle, AE is the altitude corresponding to the base BC. Now draw AD || BC through A and
DC || AB through C and let them intersect at point D. Therefore, ABCD is a parallelogram having AC as its
diagonal. We know that the diagonal of a parallelogram divides it into two congruent triangles.
\ Area of DABC = Area of DADC
Also, Area of DABC + Area of DADC = Area of the parallelogram ABCD

⇒ 2(Area of D ABC) = Area of parallelogram ABCD
1 1
⇒ Area of DABC = × (Area of parallelogram ABCD) = × Base × Height (or Altitude)
2
2
1
Hence, Area of D ABC = × Base × Altitude
2
2 × Area 2 × Area
Base of triangle = and Altitude of triangle =
Altitude Base

Area of an Equilateral Triangle
Consider a triangle ABC, whose each side is equal to a units as shown in Fig. below.
a a
Draw AE ^ BC. According to Pythagoras’ theorem, in DABE, we have,

AE = AB − BE 2
2
a
a 2 a 3 2 3 1 1
2
= a − = = a units. [AE bisects BC, so BE = BC = a ]
4 4 2 2 2
1
Hence, area of DABC triangle = × base × altitude
2
1 3 3
2
= × a × a = a sq units
2 2 4

Let’s Attempt

Example 1: Find the area of an equilateral triangle, each of whose sides is 8 cm long.

3 3 3
Solution: Area of an equilateral triangle = a = (8 cm) = × 64 cm = 27.71 cm .
2
2
2
2
4 4 4