Page 140 - Maths Skills - 7

138 Maths

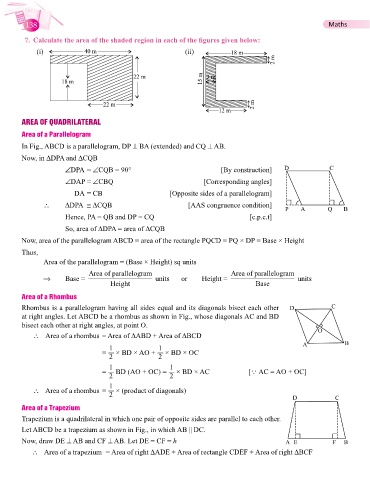
7. Calculate the area of the shaded region in each of the figures given below:

(i) (ii)

AREA OF QUADRILATERAL

Area of a Parallelogram
In Fig., ABCD is a parallelogram, DP ^ BA (extended) and CQ ^ AB.

Now, in DDPA and DCQB
∠DPA = ∠CQB = 90° [By construction]
∠DAP = ∠CBQ [Corresponding angles]

DA = CB [Opposite sides of a parallelogram]
\ DDPA @ DCQB [AAS congruence condition]

Hence, PA = QB and DP = CQ [c.p.c.t]
So, area of DDPA = area of DCQB

Now, area of the parallelogram ABCD = area of the rectangle PQCD = PQ × DP = Base × Height
Thus,
Area of the parallelogram = (Base × Height) sq units
Area of parallelogram Area of parallelogram
⇒ Base = units or Height = units
Height Base
Area of a Rhombus
Rhombus is a parallelogram having all sides equal and its diagonals bisect each other
at right angles. Let ABCD be a rhombus as shown in Fig., whose diagonals AC and BD
bisect each other at right angles, at point O.
\ Area of a rhombus = Area of DABD + Area of DBCD

1 1
= 2 × BD × AO + × BD × OC
2
1 1
= BD (AO + OC) = × BD × AC [Q AC = AO + OC]
2 2
1
\ Area of a rhombus = × (product of diagonals)
2
Area of a Trapezium
Trapezium is a quadrilateral in which one pair of opposite sides are parallel to each other.

Let ABCD be a trapezium as shown in Fig., in which AB || DC.
Now, draw DE ^ AB and CF ^ AB. Let DE = CF = h
\ Area of a trapezium = Area of right DADE + Area of rectangle CDEF + Area of right DBCF