Page 136 - Maths Skills - 7

134 Maths

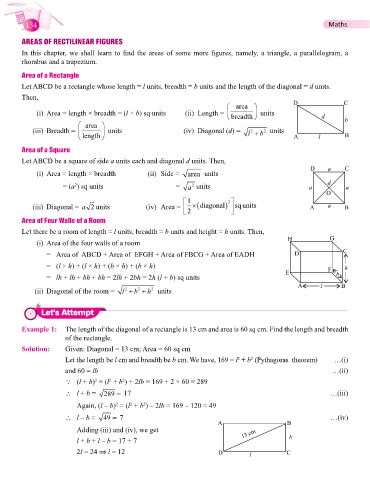
AREAS OF RECTILINEAR FIGURES
In this chapter, we shall learn to find the areas of some more figures, namely, a triangle, a parallelogram, a
rhombus and a trapezium.

Area of a Rectangle
Let ABCD be a rectangle whose length = l units, breadth = b units and the length of the diagonal = d units.
Then,
 area 
(i) Area = length × breadth = (l × b) sq units (ii) Length =   units
 breadth 
 area 
(iii) Breadth =   units (iv) Diagonal (d) = l + b 2 units
2
 length 
Area of a Square
Let ABCD be a square of side a units each and diagonal d units. Then,

(i) Area = length × breadth (ii) Side = area units
= (a ) sq units = a units
2
2
1  2 
(iii) Diagonal = a 2 units (iv) Area =  2 ×(diagonal ) sq units



Area of Four Walls of a Room
Let there be a room of length = l units, breadth = b units and height = h units. Then,
(i) Area of the four walls of a room

= Area of ABCD + Area of EFGH + Area of FBCG + Area of EADH
= (l × h) + (l × h) + (b × h) + (b × h)
= lh + lh + bh + bh = 2lh + 2bh = 2h (l + b) sq units

(ii) Diagonal of the room = l + 2 b + 2 h units
2

Let’s Attempt

Example 1: The length of the diagonal of a rectangle is 13 cm and area is 60 sq cm. Find the length and breadth
of the rectangle.
Solution: Given: Diagonal = 13 cm; Area = 60 sq cm
Let the length be l cm and breadth be b cm. We have, 169 = l + b (Pythagoras theorem) …(i)
2
2
and 60 = lb …(ii)
Q (l + b) = (l + b ) + 2lb = 169 + 2 × 60 = 289
2
2
2
\ l + b = 289 = 17 …(iii)

Again, (l – b) = (l + b ) – 2lb = 169 – 120 = 49
2
2
2
\ l – b = 49 = 7 …(iv)

Adding (iii) and (iv), we get 13 cm
l + b + l – b = 17 + 7 b
2l = 24 ⇒ l = 12