Page 141 - Maths Skills - 7

Perimeter and Area 139

1 1
= × AE × DE + EF × DE + × BF × CF
2 2
1 1 1
= × AE × h + EF × h + × BF × h = h(AE + 2EF + BF)
2 2 2
1
= 2 h(AB + EF) [Q AB = AE + EF + FB]

1
= h(AB + CD) [Q EF = CD]
2
1
\ Area of a trapezium = (sum of parallel sides) × (distance between them)
2

Let’s Attempt

Example 1: Find the area of a parallelogram one of whose sides is 20 cm and the corresponding altitude is
15 cm.

Solution: One side = 20 cm, Height = 15 cm
\ Area of parallelogram = Base × Height = 20 cm × 15 cm = 300 sq cm.

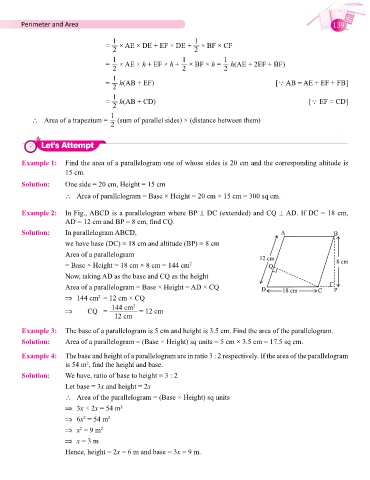
Example 2: In Fig., ABCD is a parallelogram where BP ^ DC (extended) and CQ ^ AD. If DC = 18 cm,
AD = 12 cm and BP = 8 cm, find CQ.
Solution: In parallelogram ABCD,
we have base (DC) = 18 cm and altitude (BP) = 8 cm

Area of a parallelogram
= Base × Height = 18 cm × 8 cm = 144 cm 2
Now, taking AD as the base and CQ as the height
Area of a parallelogram = Base × Height = AD × CQ
⇒ 144 cm = 12 cm × CQ
2
⇒ CQ = 144 cm 2 = 12 cm
12 cm

Example 3: The base of a parallelogram is 5 cm and height is 3.5 cm. Find the area of the parallelogram.
Solution: Area of a parallelogram = (Base × Height) sq units = 5 cm × 3.5 cm = 17.5 sq cm.

Example 4: The base and height of a parallelogram are in ratio 3 : 2 respectively. If the area of the parallelogram
is 54 m , find the height and base.
2
Solution: We have, ratio of base to height = 3 : 2

Let base = 3x and height = 2x
\ Area of the parallelogram = (Base × Height) sq units
⇒ 3x × 2x = 54 m 2
⇒ 6x = 54 m 2
2
⇒ x = 9 m 2
2
⇒ x = 3 m
Hence, height = 2x = 6 m and base = 3x = 9 m.