Page 150 - Maths Skills - 7

148 Maths

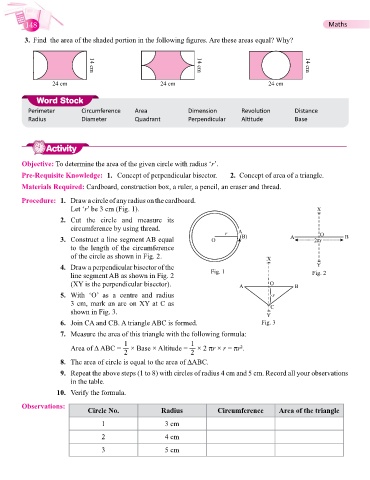
3. Find the area of the shaded portion in the following figures. Are these areas equal? Why?

24 cm 14 cm 24 cm 14 cm 24 cm 14 cm

Word Stock
Perimeter Circumference Area Dimension Revolution Distance
Radius Diameter Quadrant Perpendicular Altitude Base

Activity

Objective: To determine the area of the given circle with radius ‘r’.
Pre-Requisite Knowledge: 1. Concept of perpendicular bisector. 2. Concept of area of a triangle.

Materials Required: Cardboard, construction box, a ruler, a pencil, an eraser and thread.
Procedure: 1. Draw a circle of any radius on the cardboard.
Let ‘r’ be 3 cm (Fig. 1).
2. Cut the circle and measure its
circumference by using thread.
3. Construct a line segment AB equal
to the length of the circumference
of the circle as shown in Fig. 2.
4. Draw a perpendicular bisector of the Fig. 1
line segment AB as shown in Fig. 2 Fig. 2
(XY is the perpendicular bisector).
5. With ‘O’ as a centre and radius
3 cm, mark an arc on XY at C as
shown in Fig. 3.

6. Join CA and CB. A triangle ABC is formed. Fig. 3
7. Measure the area of this triangle with the following formula:
1 1
Area of D ABC = × Base × Altitude = × 2 pr × r = pr .
2
2 2
8. The area of circle is equal to the area of DABC.
9. Repeat the above steps (1 to 8) with circles of radius 4 cm and 5 cm. Record all your observations
in the table.
10. Verify the formula.

Observations:
Circle No. Radius Circumference Area of the triangle
1 3 cm

2 4 cm

3 5 cm