Page 77 - Maths Skill - 6

Fractions 75

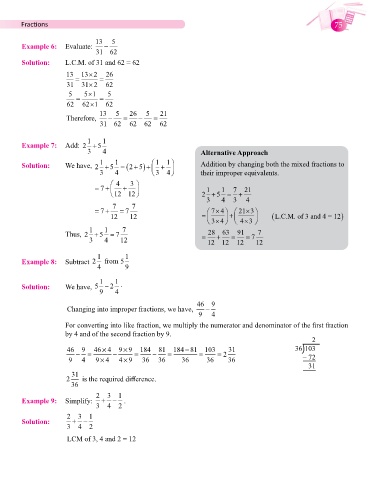
Example 6: Evaluate: 13 - 5
31 62
Solution: L.C.M. of 31 and 62 = 62

13 13 2 26

31 31 2 62

5 51 5

62 62 1 62
13 5 26 5 5 21
Therefore,
31 62 62 62 62
1
Example 7: Add: 2 + 5 1
3 4 Alternative Approach
1
1
Solution: We have, 2 5 25 1 1 Addition by changing both the mixed fractions to

3 4 3 4 their improper equivalents.
4 3 1 1 7 21

7
12 12 2 5
3 4 3 4
7 7 7 74 21 3

7

12 12 L.C.M. of 3 and 4 = 12

34 43
1
1
Thus, 2 5 7 7 28 63 91 7 7
3 4 12 12 12 12 12
1 1
Example 8: Subtract 2 from 5
4 9
1
1
Solution: We have, 5 - 2 ·
9 4
Changing into improper fractions, we have, 46 - 9
9 4
For converting into like fraction, we multiply the numerator and denominator of the first fraction
by 4 and of the second fraction by 9.
2

46 − 9 = 46 4× − 99× = 184 − 81 = 184 81 103− = = 2 31 36 103
9 4 94× 49× 36 36 36 36 36 – 72
31
2 31 is the required difference.
36
3
2
Example 9: Simplify: 1 .
3 4 2
3
Solution: 2 1
3 4 2

LCM of 3, 4 and 2 = 12