Page 74 - Maths Skill - 6

72 Maths

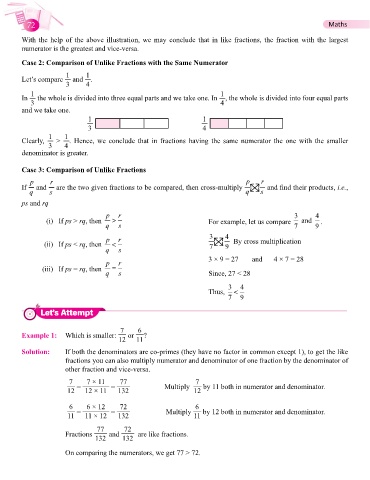
With the help of the above illustration, we may conclude that in like fractions, the fraction with the largest
numerator is the greatest and vice-versa.

Case 2: Comparison of Unlike Fractions with the Same Numerator
1 1
Let’s compare and .
3 4
1 1
In the whole is divided into three equal parts and we take one. In , the whole is divided into four equal parts
3
4
and we take one.
1 1
3 4
1 1
Clearly, > . Hence, we conclude that in fractions having the same numerator the one with the smaller
3 4
denominator is greater.

Case 3: Comparison of Unlike Fractions
p r p r
If and are the two given fractions to be compared, then cross-multiply and find their products, i.e.,
q s q s
ps and rq
p r 3 4
(i) If ps > rq, then > For example, let us compare and .
q s 7 9
p r 3 4 By cross multiplication
(ii) If ps < rq, then < 7 9
q s
3 × 9 = 27 and 4 × 7 = 28
p r
(iii) If ps = rq, then =
q s Since, 27 < 28

Thus, 3 < 4
7 9

Let’s Attempt

7 6
Example 1: Which is smaller: or ?
12 11

Solution: If both the denominators are co-primes (they have no factor in common except 1), to get the like
fractions you can also multiply numerator and denominator of one fraction by the denominator of
other fraction and vice-versa.

7 7 × 11 77 7
12 = 12 × 11 = 132 Multiply 12 by 11 both in numerator and denominator.

6 6 × 12 72 6
11 = 11 × 12 = 132 Multiply 11 by 12 both in numerator and denominator.

77 72
Fractions and are like fractions.
132 132
On comparing the numerators, we get 77 > 72.