Page 73 - Maths Skill - 6

Fractions 71

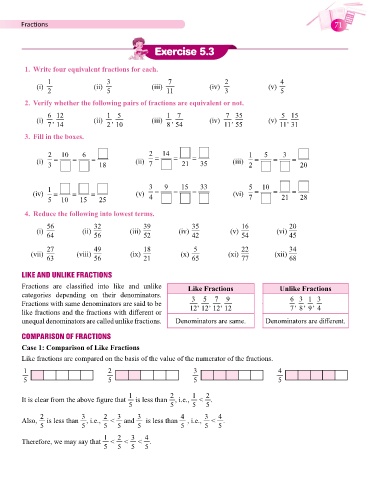
Exercise 5.3

1. Write four equivalent fractions for each.

1 3 7 2 4
(i) (ii) (iii) (iv) (v)
2 5 11 3 5
2. Verify whether the following pairs of fractions are equivalent or not.

6 12 1 5 1 7 7 35 5 15
(i) 7 14 (ii) 2 10 (iii) 8 54 (iv) 11 55 (v) 11 31
,
,
,
,
,
3. Fill in the boxes.
2 10 6 2 = 14 = = 1 5 3
(i) = = = (ii) (iii) = = =
3 18 7 21 35 2 20

(iv) 1 = = = (v) 3 = 9 = 15 = 33 (vi) 5 = 10 = =
5 10 15 25 4 7 21 28
4. Reduce the following into lowest terms.

56 32 39 35 16 20
(i) (ii) (iii) (iv) (v) (vi)
64 56 52 42 54 45
27 49 18 5 22 34
(vii) (viii) (ix) (x) (xi) (xii)
63 56 21 65 77 68

LIKE AND UNLIKE FRACTIONS
Fractions are classified into like and unlike Like Fractions Unlike Fractions
categories depending on their denominators. 3 5 7 9 6 3 1 3
Fractions with same denominators are said to be , , , , , ,
like fractions and the fractions with different or 12 12 12 12 7 8 9 4
unequal denominators are called unlike fractions. Denominators are same. Denominators are different.

COMPARISON OF FRACTIONS

Case 1: Comparison of Like Fractions
Like fractions are compared on the basis of the value of the numerator of the fractions.
1 2 3 4
5 5 5 5

1 2 1 2
It is clear from the above figure that is less than , i.e., < .
5 5 5 5

2 3 2 3 3 4 3 4
Also, is less than , i.e., < and is less than , i.e., < .
5 5 5 5 5 5 5 5
1 2 3 4
Therefore, we may say that < < < .
5 5 5 5