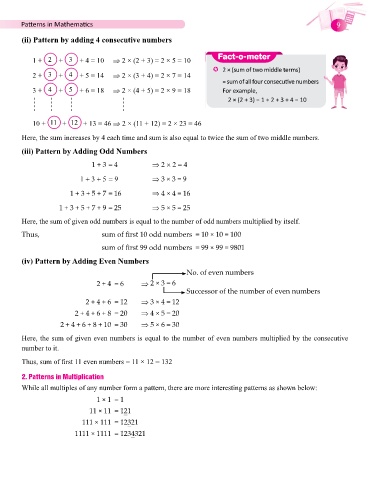
Page 11 - Maths Skill - 6

Patterns in Mathematics 9

(ii) Pattern by adding 4 consecutive numbers

1 + 2 + 3 + 4 = 10 ⇒ 2 × (2 + 3) = 2 × 5 = 10 Fact-o-meter
� 2 × (sum of two middle terms)
2 + 3 + 4 + 5 = 14 ⇒ 2 × (3 + 4) = 2 × 7 = 14
= sum of all four consecutive numbers
3 + 4 + 5 + 6 = 18 ⇒ 2 × (4 + 5) = 2 × 9 = 18 For example,
2 × (2 + 3) = 1 + 2 + 3 + 4 = 10

10 + 11 + 12 + 13 = 46 ⇒ 2 × (11 + 12) = 2 × 23 = 46

Here, the sum increases by 4 each time and sum is also equal to twice the sum of two middle numbers.

(iii) Pattern by Adding Odd Numbers

1 + 3 = 4 ⇒ 2 × 2 = 4

1 + 3 + 5 = 9 ⇒ 3 × 3 = 9

1 + 3 + 5 + 7 = 16 ⇒ 4 × 4 = 16

1 + 3 + 5 + 7 + 9 = 25 ⇒ 5 × 5 = 25

Here, the sum of given odd numbers is equal to the number of odd numbers multiplied by itself.
Thus, sum of first 10 odd numbers = 10 × 10 = 100

sum of first 99 odd numbers = 99 × 99 = 9801

(iv) Pattern by Adding Even Numbers
No. of even numbers
2 + 4 = 6 ⇒ 2 × 3 = 6
Successor of the number of even numbers
2 + 4 + 6 = 12 ⇒ 3 × 4 = 12

2 + 4 + 6 + 8 = 20 ⇒ 4 × 5 = 20
2 + 4 + 6 + 8 + 10 = 30 ⇒ 5 × 6 = 30

Here, the sum of given even numbers is equal to the number of even numbers multiplied by the consecutive
number to it.

Thus, sum of first 11 even numbers = 11 × 12 = 132

2. Patterns in Multiplication
While all multiples of any number form a pattern, there are more interesting patterns as shown below:
1 × 1 = 1

11 × 11 = 121
111 × 111 = 12321
1111 × 1111 = 1234321