Page 10 - Maths Skill - 6

8 Maths

INTRODUCTION
Basically mathematics is all about numbers. It also involves the study of different patterns. There are different
types of patterns such as number patterns, image patterns, word patterns etc. Number patterns are very common
in mathematics. There are quite familiar to the students who study maths frequently. Especially number patterns
are everywhere in mathematics. Apart from this, nature is the best example which shows patterns. Nature provides
patterns in flowers, animals and shapes. The secret of discovering these patterns is to look around. This is called
observations.
Numbers are facinating and so are the patterns.

PATTERNS IN NUMBERS
Numbers have fascinated humans since ages, be it the mathematicians or statisticians. There is so much that one
can do with them, and there’s so much that is yet to be discovered, for example, we know that the whole numbers
represent the set of all positive numbers. Including zero without any decimal or fractional part. But did we know
that we can derive relationships between the whole numbers by finding some kind of patterns between them.
Let’s explore some kind of sequences that are studied in mathematics.
(i) Even number Series : 0, 2, 4, 6, 8, 10, 12 ...

(ii) Odd number Series : 1, 3, 5, 7, 9, 11 ...
(iii) Counting Number Series : 1, 2, 3, 4, 5, 6, 7, 8 ...

(iv) Triangular Number Series : 1, 3, 6, 10, 15 ...
(v) Square Number Series : 1, 4, 9, 16, 25, 36 ...
(vi) Cube Number Series : 1, 8, 27, 64, 125 ...

(vii) Fibonacci Number Series : 0, 1, 1, 2, 3, 5, 8, 13, 21 ...
and many more.

VISUALISING NUMBER SEQUENCES
Here, we will study about number patterns which have been explained in previous topic.

1. Patterns in Addition
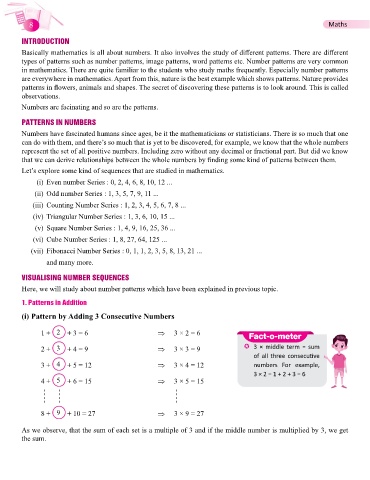
(i) Pattern by Adding 3 Consecutive Numbers

1 + 2 + 3 = 6 ⇒ 3 × 2 = 6 Fact-o-meter

2 + 3 + 4 = 9 ⇒ 3 × 3 = 9 � 3 × middle term = sum
of all three consecutive
3 + 4 + 5 = 12 ⇒ 3 × 4 = 12 numbers For example,
3 × 2 = 1 + 2 + 3 = 6
4 + 5 + 6 = 15 ⇒ 3 × 5 = 15

8 + 9 + 10 = 27 ⇒ 3 × 9 = 27

As we observe, that the sum of each set is a multiple of 3 and if the middle number is multiplied by 3, we get
the sum.