Page 13 - Maths Skill - 6

Patterns in Mathematics 11

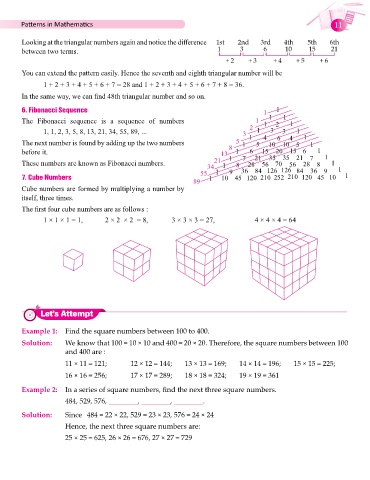
Looking at the triangular numbers again and notice the difference 1st 2nd 3rd 4th 5th 6th
between two terms. 1 3 6 10 15 21
+ 2 + 3 + 4 + 5 + 6
You can extend the pattern easily. Hence the seventh and eighth triangular number will be
1 + 2 + 3 + 4 + 5 + 6 + 7 = 28 and 1 + 2 + 3 + 4 + 5 + 6 + 7 + 8 = 36.

In the same way, we can find 48th triangular number and so on.

6. Fibonacci Sequence 1 1
The Fibonacci sequence is a sequence of numbers 1 1 1 2 1 1
1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, ... 3 2 1 3 3 1
4
The next number is found by adding up the two numbers 5 1 1 5 4 10 6 10 5 1 1
before it. 13 8 1 6 15 20 15 6 1
35
These numbers are known as Fibonacci numbers. 21 1 7 21 56 70 35 21 28 7 8 1 1
56
34 1 8 36 28 84 126 126 84 36 9 1
9
7. Cube Numbers 55 1 1 10 45 120 210 252 210 120 45 10 1
89
Cube numbers are formed by multiplying a number by
itself, three times.
The first four cube numbers are as follows :
1 × 1 × 1 = 1, 2 × 2 × 2 = 8, 3 × 3 × 3 = 27, 4 × 4 × 4 = 64

Let’s Attempt

Example 1: Find the square numbers between 100 to 400.

Solution: We know that 100 = 10 × 10 and 400 = 20 × 20. Therefore, the square numbers between 100
and 400 are :
11 × 11 = 121; 12 × 12 = 144; 13 × 13 = 169; 14 × 14 = 196; 15 × 15 = 225;
16 × 16 = 256; 17 × 17 = 289; 18 × 18 = 324; 19 × 19 = 361

Example 2: In a series of square numbers, find the next three square numbers.

484, 529, 576, ________, ________, ________.
Solution: Since 484 = 22 × 22, 529 = 23 × 23, 576 = 24 × 24

Hence, the next three square numbers are:
25 × 25 = 625, 26 × 26 = 676, 27 × 27 = 729