Page 58 - Mathematics Class - XI

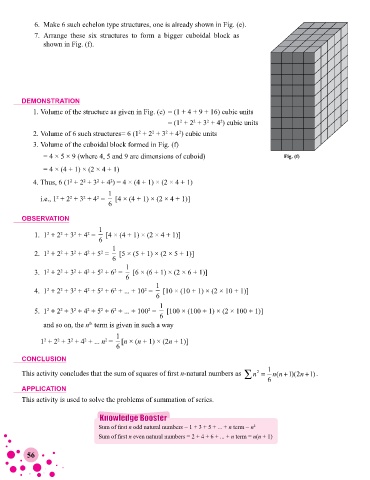
6. Make 6 such echelon type structures, one is already shown in Fig. (e).
7. Arrange these six structures to form a bigger cuboidal block as
shown in Fig. (f).

DEMONSTRATION
1. Volume of the structure as given in Fig. (e) = (1 + 4 + 9 + 16) cubic units
= (1 + 2 + 3 + 4 ) cubic units
2
2
2
2
2. Volume of 6 such structures = 6 (1 + 2 + 3 + 4 ) cubic units
2
2
2
2
3. Volume of the cuboidal block formed in Fig. (f)
= 4 × 5 × 9 (where 4, 5 and 9 are dimensions of cuboid) Fig. (f)
= 4 × (4 + 1) × (2 × 4 + 1)
4. Thus, 6 (1 + 2 + 3 + 4 ) = 4 × (4 + 1) × (2 × 4 + 1)
2
2
2
2
1
i.e., 1 + 2 + 3 + 4 = [4 × (4 + 1) × (2 × 4 + 1)]
2
2
2
2
6
OBSERVATION
1
1. 1 + 2 + 3 + 4 = [4 × (4 + 1) × (2 × 4 + 1)]
2
2
2
2
6
1
2. 1 + 2 + 3 + 4 + 5 = [5 × (5 + 1) × (2 × 5 + 1)]
2
2
2
2
2
6
1
3. 1 + 2 + 3 + 4 + 5 + 6 = [6 × (6 + 1) × (2 × 6 + 1)]
2
2
2
2
2
2
6
1
4. 1 + 2 + 3 + 4 + 5 + 6 + ... + 10 = [10 × (10 + 1) × (2 × 10 + 1)]
2
2
2
2
2
2
2
6
1
5. 1 + 2 + 3 + 4 + 5 + 6 + ... + 100 = [100 × (100 + 1) × (2 × 100 + 1)]
2
2
2
2
2
2
2
6
and so on, the n term is given in such a way
th
1
1 + 2 + 3 + 4 + ... n = [n × (n + 1) × (2n + 1)]
2
2
2
2
2
6
CONCLUSION
This activity concludes that the sum of squares of first n-natural numbers as ∑ n = 1 n n 12+( )( n 1+ ) .
2
6
APPLICATION
This activity is used to solve the problems of summation of series.
Knowledge Booster
Sum of first n odd natural numbers = 1 + 3 + 5 + ... + n term = n 2
Sum of first n even natural numbers = 2 + 4 + 6 + ... + n term = n(n + 1)
56