Page 60 - Mathematics Class - XI

ACTIVITY 8.2

OBJECTIVE
To demonstrate that the Arithmetic mean of two different positive numbers is always greater than the Geometric
mean.

MATERIAL REQUIRED
 Cardboard  Cutter
 White chart paper  Scale
 Coloured chart paper  Glue

 Sketch pens

PRE-REQUISITE KNOWLEDGE
1. Knowledge of arithmetic progression and geometric progression
2. Knowledge of arithmetic mean and geometric mean

PROCEDURE
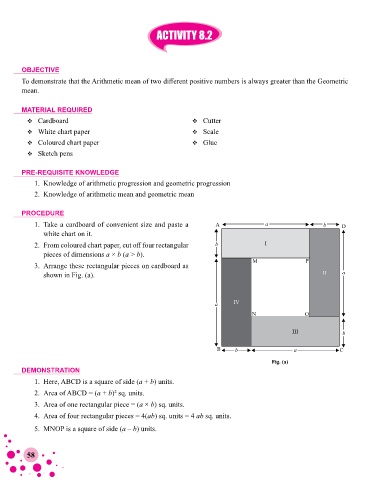
1. Take a cardboard of convenient size and paste a
white chart on it.

2. From coloured chart paper, cut off four rectangular
pieces of dimensions a × b (a > b).

3. Arrange these rectangular pieces on cardboard as
shown in Fig. (a).

Fig. (a)
DEMONSTRATION
1. Here, ABCD is a square of side (a + b) units.
2. Area of ABCD = (a + b) sq. units.
2
3. Area of one rectangular piece = (a × b) sq. units.

4. Area of four rectangular pieces = 4(ab) sq. units = 4 ab sq. units.
5. MNOP is a square of side (a – b) units.

58