Page 61 - Mathematics Class - XI

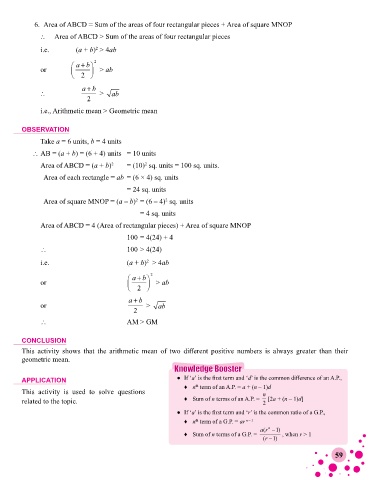
6. Area of ABCD = Sum of the areas of four rectangular pieces + Area of square MNOP
⸫ Area of ABCD > Sum of the areas of four rectangular pieces

i.e. (a + b) > 4ab
2
ab+ 
or    2   2 > ab

ab+
⸫ > ab
2

i.e., Arithmetic mean > Geometric mean

OBSERVATION
Take a = 6 units, b = 4 units

\ AB = (a + b) = (6 + 4) units = 10 units
Area of ABCD = (a + b) = (10) sq. units = 100 sq. units.
2
2
Area of each rectangle = ab = (6 × 4) sq. units
= 24 sq. units
Area of square MNOP = (a – b) = (6 – 4) sq. units
2
2
= 4 sq. units
Area of ABCD = 4 (Area of rectangular pieces) + Area of square MNOP

100 = 4(24) + 4
\ 100 > 4(24)

i.e. (a + b) > 4ab
2
ab+ 
or    2   2 > ab

ab+
or > ab
2
\ AM > GM

CONCLUSION
This activity shows that the arithmetic mean of two different positive numbers is always greater than their
geometric mean.
Knowledge Booster

APPLICATION ● If ‘a’ is the first term and ‘d’ is the common difference of an A.P.,
♦ n term of an A.P. = a + (n – 1)d
th
This activity is used to solve questions n
related to the topic. ♦ Sum of n terms of an A.P. = 2 [2a + (n – 1)d]
● If ‘a’ is the first term and ‘r’ is the common ratio of a G.P.,
♦ n term of a G.P. = ar n – 1
th
ar( n −1 )
♦ Sum of n terms of a G.P. = , when r > 1
r ( −1 )

59