Page 27 - Mathematics Class - XI

TOPIC - 3: Trigonometric Functions

ACTIVITY 3.1

OBJECTIVE
To verify the relation between the degree measure and the radian measure of an angle.

MATERIAL REQUIRED
 Cardboard  White chart paper  Threads or yarns
 Geometry box  Bangle  Glue

PRE-REQUISITE KNOWLEDGE
1. Knowledge of angles, circle and length of arc 2. Knowledge of measurement of angle

PROCEDURE
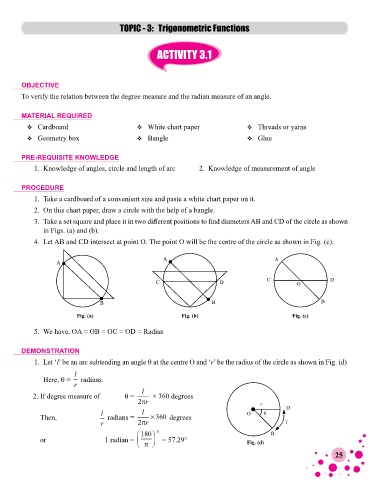
1. Take a cardboard of a convenient size and paste a white chart paper on it.
2. On this chart paper, draw a circle with the help of a bangle.

3. Take a set square and place it in two different positions to find diameters AB and CD of the circle as shown
in Figs. (a) and (b).

4. Let AB and CD intersect at point O. The point O will be the centre of the circle as shown in Fig. (c).

A A
A

C D C O D

B B B
Fig. (a) Fig. (b) Fig. (c)

5. We have, OA = OB = OC = OD = Radius

DEMONSTRATION
1. Let ‘l’ be an arc subtending an angle q at the centre O and ‘r’ be the radius of the circle as shown in Fig. (d)
l
Here, q = radians.
r

2. If degree measure of q = l × 360 degrees
2p r r
l l O q D
Then, radians = × 360 degrees
r 2π r l

 180 ° B
or 1 radian =  π   = 57.29° Fig. (d)

25