Page 32 - Mathematics Class - XI

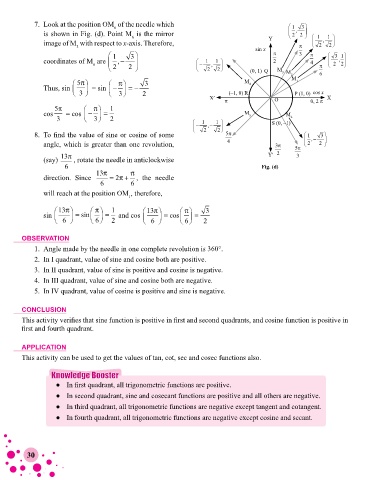
7. Look at the position OM of the needle which  1 3 
6
,
is shown in Fig. (d). Point M is the mirror   2 2    1 1 
6
image of M with respect to x-axis. Therefore, Y   , 2  
3 sin x p 2
 1 3  p 3 p  3 1 
coordinates of M are   2 ,− 2      − 1 2 , 1 2    (0, 1) Q 2 M 4 p   2 2 
,
6
 5π  π  3 M 4 3 M 2 M 1 6
Thus, sin   = sin −  =−

 3   3  2 (–1, 0) R P (1, 0) cos x
X′ X
p O 0, 2 p
5p  π  1
cos = cos −  = M 5 M

3  3  2  1 1  S (0, –1) 6
  − − ,  
8. To find the value of sine or cosine of some 2 2 5p  1 ,− 3 
angle, which is greater than one revolution, 4 3p 5p   2 2  

(say) 13p , rotate the needle in anticlockwise Y′ 2 3
6 Fig. (d)
direction. Since 13π = 2π + π , the needle
6 6
will reach at the position OM , therefore,
1
 13π  π 1  13π  π 3
sin   = sin   = and cos   = cos   =
 6   6  2  6   6  2

OBSERVATION
1. Angle made by the needle in one complete revolution is 360°.
2. In I quadrant, value of sine and cosine both are positive.

3. In II quadrant, value of sine is positive and cosine is negative.
4. In III quadrant, value of sine and cosine both are negative.
5. In IV quadrant, value of cosine is positive and sine is negative.

CONCLUSION
This activity verifies that sine function is positive in first and second quadrants, and cosine function is positive in
first and fourth quadrant.

APPLICATION
This activity can be used to get the values of tan, cot, sec and cosec functions also.

Knowledge Booster
● In first quadrant, all trigonometric functions are positive.
● In second quadrant, sine and cosecant functions are positive and all others are negative.

● In third quadrant, all trigonometric functions are negative except tangent and cotangent.
● In fourth quadrant, all trigonometric functions are negative except cosine and secant.

30