Page 30 - Mathematics Class - XI

ACTIVITY 3.2

OBJECTIVE
To find the values of sine and cosine functions in second, third and fourth quadrants using their given values in
the first quadrant.

MATERIAL REQUIRED
 Cardboard  Geometry box  Steel wires
 White chart paper  Glue  Needle

PRE-REQUISITE KNOWLEDGE
1. Knowledge of coordinate system and quadrants 2. Knowledge of trigonometric functions

PROCEDURE

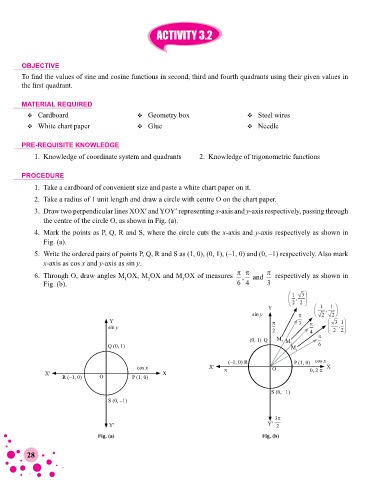
1. Take a cardboard of convenient size and paste a white chart paper on it.
2. Take a radius of 1 unit length and draw a circle with centre O on the chart paper.
3. Draw two perpendicular lines XOX′ and YOY′ representing x-axis and y-axis respectively, passing through
the centre of the circle O, as shown in Fig. (a).

4. Mark the points as P, Q, R and S, where the circle cuts the x-axis and y-axis respectively as shown in
Fig. (a).
5. Write the ordered pairs of points P, Q, R and S as (1, 0), (0, 1), (–1, 0) and (0, –1) respectively. Also mark
x-axis as cos x and y-axis as sin y.

6. Through O, draw angles M OX, M OX and M OX of measures pp p respectively as shown in
, and
3
1
2
Fig. (b). 64 3
 1 3 
,
  2 2  
Y  1 1 
sin y p   2 , 2  
Y p 3 p  3 1 
sin y  , 
2 4  2 2
(0, 1) Q M 3 M p
Q (0, 1) 2 M 6
1
(–1, 0) R P (1, 0) cos x
cos x X′ p O 0, 2 p X
X′ X
R (–1, 0) O P (1, 0)

S (0, –1)
S (0, –1)

3p
Y′ Y′ 2
Fig. (a) Fig. (b)

28