Page 31 - Mathematics Class - XI

7. Now, take a needle of unit length. Fix one end of it at the centre of circle and the other end to move freely
along the circle.

DEMONSTRATION

 3 1  p p
1. The coordinates of the point M are   2 2  because its x-coordinate is cos and y-coordinate is sin .
,
1
6
6
 1 1   1 3 
Similarly, the coordinates of the point M and M are  2 , 2   and  2 2  .
,

3
2


2. To find the value of sine or cosine of some angle in the II quadrant (say) 3p , rotate the needle in anticlockwise
4
3p
direction making an angle M OX of measure = 135° with the positive direction of x-axis.
4
4
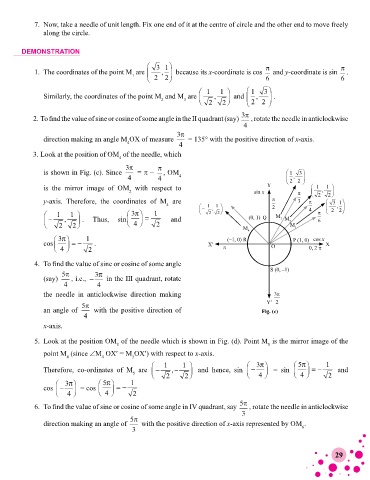
3. Look at the position of OM of the needle, which
4
3p p
is shown in Fig. (c). Since = p – , OM  1 3 
,
4 4 4   2 2  
is the mirror image of OM with respect to Y  1 1 
2 sin x p   2 , 2  
y-axis. Therefore, the coordinates of M are p 3 p  3 1 
4  − 1 , 1  2 4  , 
 1 1   3π 1   2 2   (0, 1) Q M p  2 2

  − ,   . Thus, sin   4  = 2 and 3 M 2 M 6
2
2
M
4 1
cos    3π =− 1 2 . X′ p (–1, 0) R O P (1, 0) cos x X

4 
0, 2 p
4. To find the value of sine or cosine of some angle
5p 3π S (0, –1)
(say) , i.e., − in the III quadrant, rotate
4 4
the needle in anticlockwise direction making 3p
5p Y′ 2
an angle of with the positive direction of Fig. (c)
4
x-axis.

5. Look at the position OM of the needle which is shown in Fig. (d). Point M is the mirror image of the
5
5
point M (since ∠M OX′ = M OX′) with respect to x-axis.
4 4 5
 1 1   3π   5π 1
Therefore, co-ordinates of M are − − ,  and hence, sin −  = sin   =− and


5  2 2   4   4  2
 3π   5π 1
cos − 4  = cos  4  =− 2





6. To find the value of sine or cosine of some angle in IV quadrant, say 5p , rotate the needle in anticlockwise
3
direction making an angle of 5p with the positive direction of x-axis represented by OM .
3 6

29