Page 19 - Mathematics Class - XI

TOPIC - 2: Relations and Functions

ACTIVITY 2.1

OBJECTIVE
To verify that for two sets A and B, n (A × B) = pq and the total number of relations from A to B is 2 , where
pq
n (A) = p and n (B) = q.

MATERIAL REQUIRED
 White paper
 Pen/Pencil

PRE-REQUISITE KNOWLEDGE
1. Knowledge of sets

2. Knowledge of relations of sets

PROCEDURE
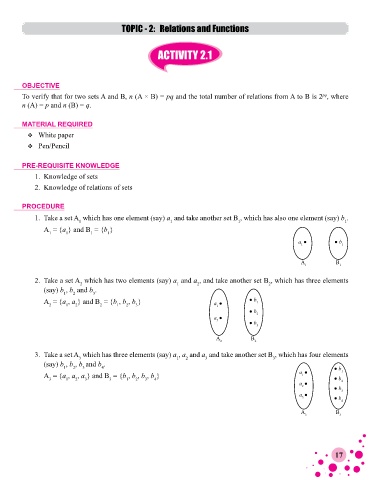
1. Take a set A which has one element (say) a and take another set B , which has also one element (say) b .
1 1 1 1
A = {a } and B = {b }
1
1
1
1
a ● ● b 1
1
A 1 B 1

2. Take a set A which has two elements (say) a and a , and take another set B , which has three elements
2
1
2
2
(say) b , b and b .
1 2 3
A = {a , a } and B = {b , b , b } a ● ● b 1
2 1 2 2 1 2 3 1
● b 2
a ●
2
● b 3
A B
2 2
3. Take a set A which has three elements (say) a , a and a and take another set B , which has four elements
3
3
1
3
2
(say) b , b , b and b . ● b
2
1
4
3
A = {a , a , a } and B = {b , b , b , b } a ● 1
1
3 1 2 3 3 1 2 3 4 ● b 2
a ● ● b
2
a ● 3
3 ● b 4
A 3 B 3

17