Page 12 - Mathematics Class - XI

ACTIVITY 1.2

OBJECTIVE
To represent set theoretic operations using Venn diagrams.

MATERIAL REQUIRED
 A piece of cardboard  White thick sheets of paper  Pencil
 A pair of scissors  Adhesive

PRE-REQUISITE KNOWLEDGE
1. Knowledge of sets 3. Knowledge of operations on sets
2. Knowledge of Venn diagrams

PROCEDURE
1. Cut rectangular strips from a sheet of paper and paste them on a cardboard.
2. Write ‘U’ in the left/right top corner of each rectangular strip.
3. Draw circles A and B inside each of the rectangular strips and shade different portions as shown in
Figs. (a) to (k).

DEMONSTRATION
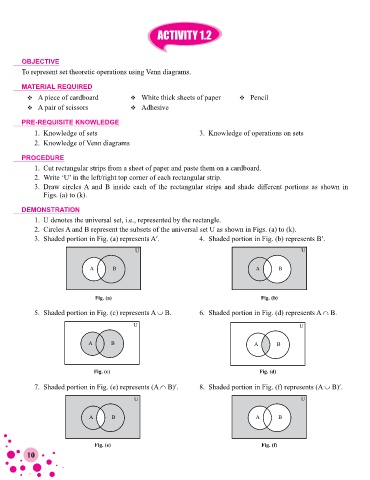
1. U denotes the universal set, i.e., represented by the rectangle.
2. Circles A and B represent the subsets of the universal set U as shown in Figs. (a) to (k).
3. Shaded portion in Fig. (a) represents A′. 4. Shaded portion in Fig. (b) represents B′.

U U

A B A B

Fig. (a) Fig. (b)
5. Shaded portion in Fig. (c) represents A ∪ B. 6. Shaded portion in Fig. (d) represents A ∩ B.

U U

A B A B

Fig. (c) Fig. (d)

7. Shaded portion in Fig. (e) represents (A ∩ B)′. 8. Shaded portion in Fig. (f) represents (A ∪ B)′.

U U

A B A B

Fig. (e) Fig. (f)
10