Page 10 - Mathematics Class - XI

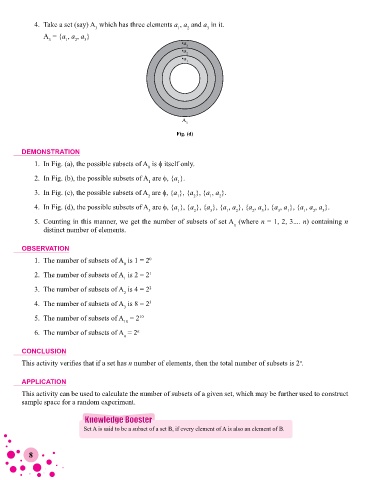
4. Take a set (say) A which has three elements a , a and a in it.
3 1 2 3
A = {a , a , a }
1
3
3
2
•a 3
•a 2
•a
1

A
3
Fig. (d)

DEMONSTRATION

1. In Fig. (a), the possible subsets of A is φ itself only.
0
2. In Fig. (b), the possible subsets of A are φ, {a }.
1 1
3. In Fig. (c), the possible subsets of A are φ, {a }, {a }, {a , a }.
2
2
1
1
2
4. In Fig. (d), the possible subsets of A are φ, {a }, {a }, {a }, {a , a }, {a , a }, {a , a }, {a , a , a }.
3 1 2 3 1 2 2 3 3 1 1 2 3
5. Counting in this manner, we get the number of subsets of set A (where n = 1, 2, 3.... n) containing n
n
distinct number of elements.
OBSERVATION

1. The number of subsets of A is 1 = 2 0
0
2. The number of subsets of A is 2 = 2 1
1
3. The number of subsets of A is 4 = 2 2
2
4. The number of subsets of A is 8 = 2 3
3
5. The number of subsets of A = 2 10
10
6. The number of subsets of A = 2 n
n

CONCLUSION
This activity verifies that if a set has n number of elements, then the total number of subsets is 2 .
n

APPLICATION
This activity can be used to calculate the number of subsets of a given set, which may be further used to construct
sample space for a random experiment.

Knowledge Booster

Set A is said to be a subset of a set B, if every element of A is also an element of B.

8