Page 107 - Maths Skills - 8

Linear Equations in One Variable 105

⇒ x + 23 = 2x – 30

⇒ x – 2x = – 30 – 23
⇒ – x = – 53 Hence, present age of Rajesh = x + 8 = 53 + 8 = 61 years,
⇒ x = 53 and present age of Sailesh = x = 53 years.

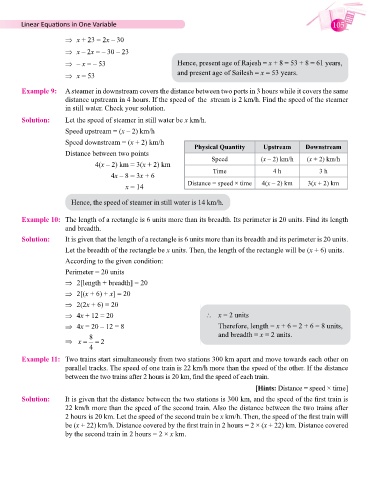
Example 9: A steamer in downstream covers the distance between two ports in 3 hours while it covers the same
distance upstream in 4 hours. If the speed of the stream is 2 km/h. Find the speed of the steamer
in still water. Check your solution.

Solution: Let the speed of steamer in still water be x km/h.
Speed upstream = (x – 2) km/h
Speed downstream = (x + 2) km/h Physical Quantity Upstream Downstream

Distance between two points
4(x – 2) km = 3(x + 2) km Speed (x – 2) km/h (x + 2) km/h
4x – 8 = 3x + 6 Time 4 h 3 h

x = 14 Distance = speed × time 4(x – 2) km 3(x + 2) km

Hence, the speed of steamer in still water is 14 km/h.

Example 10: The length of a rectangle is 6 units more than its breadth. Its perimeter is 20 units. Find its length
and breadth.
Solution: It is given that the length of a rectangle is 6 units more than its breadth and its perimeter is 20 units.
Let the breadth of the rectangle be x units. Then, the length of the rectangle will be (x + 6) units.

According to the given condition:
Perimeter = 20 units
⇒ 2[length + breadth] = 20

⇒ 2[(x + 6) + x] = 20
⇒ 2(2x + 6) = 20
⇒ 4x + 12 = 20 ∴ x = 2 units
⇒ 4x = 20 – 12 = 8 Therefore, length = x + 6 = 2 + 6 = 8 units,
and breadth = x = 2 units.
⇒ x = 8 = 2

4
Example 11: Two trains start simultaneously from two stations 300 km apart and move towards each other on
parallel tracks. The speed of one train is 22 km/h more than the speed of the other. If the distance
between the two trains after 2 hours is 20 km, find the speed of each train.
[Hints: Distance = speed × time]
Solution: It is given that the distance between the two stations is 300 km, and the speed of the first train is
22 km/h more than the speed of the second train. Also the distance between the two trains after
2 hours is 20 km. Let the speed of the second train be x km/h. Then, the speed of the first train will
be (x + 22) km/h. Distance covered by the first train in 2 hours = 2 × (x + 22) km. Distance covered
by the second train in 2 hours = 2 × x km.