Page 102 - Maths Skills - 8

100 Maths

EQUATIONS REDUCIBLE TO LINEAR FORM
To reduce a given equation to linear form, you need to cross-multiply its terms on both sides of equality. After
cross-multiplication, you will get an equation involving variables having power more than one. But on simplifying,
the terms with power more than one, like x , cancel out and you are left with a linear equation which you can solve
2
in the usual manner.

You can learn more by solving the examples given below:

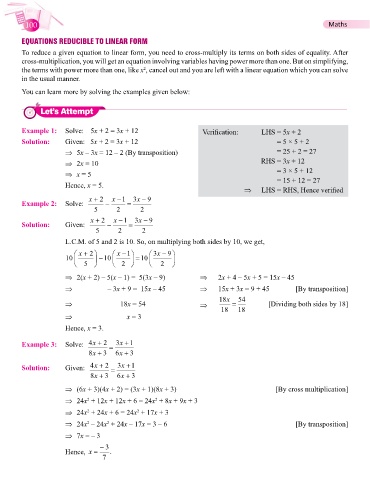
Let’s Attempt

Example 1: Solve: 5x + 2 = 3x + 12 Verification: LHS = 5x + 2
Solution: Given: 5x + 2 = 3x + 12 = 5 × 5 + 2
⇒ 5x – 3x = 12 – 2 (By transposition) = 25 + 2 = 27

⇒ 2x = 10 RHS = 3x + 12
⇒ x = 5 = 3 × 5 + 12
Hence, x = 5. = 15 + 12 = 27
⇒ LHS = RHS, Hence verified

Example 2: Solve: x 2 x 1 3 x 9
5 2 2
Solution: Given: x 2 x 1 3 x 9
5 2 2
L.C.M. of 5 and 2 is 10. So, on multiplying both sides by 10, we get,
x 2 x 1 3x
9
10 10 10
5 2 2
⇒ 2(x + 2) – 5(x – 1) = 5(3x – 9) ⇒ 2x + 4 – 5x + 5 = 15x – 45
⇒ – 3x + 9 = 15x – 45 ⇒ 15x + 3x = 9 + 45 [By transposition]

18x 54
⇒ 18x = 54 ⇒ = [Dividing both sides by 18]
⇒ x = 3 18 18

Hence, x = 3.

Example 3: Solve: 4x 2 3x 1
8x 3 6x 3

Solution: Given: 4x 2 3x 1
8x 3 6x 3
⇒ (6x + 3)(4x + 2) = (3x + 1)(8x + 3) [By cross multiplication]

⇒ 24x + 12x + 12x + 6 = 24x + 8x + 9x + 3
2
2
⇒ 24x + 24x + 6 = 24x + 17x + 3
2
2
⇒ 24x – 24x + 24x – 17x = 3 – 6 [By transposition]
2
2
⇒ 7x = – 3

Hence, x 3 .
7