Page 106 - Maths Skills - 8

104 Maths

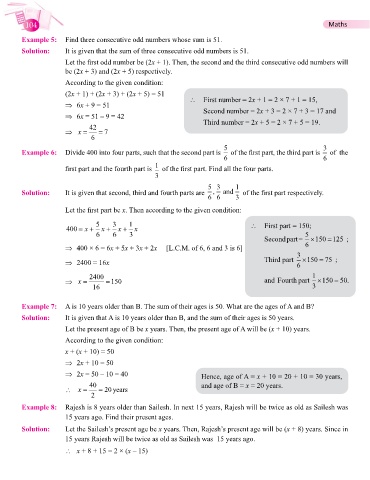
Example 5: Find three consecutive odd numbers whose sum is 51.
Solution: It is given that the sum of three consecutive odd numbers is 51.

Let the first odd number be (2x + 1). Then, the second and the third consecutive odd numbers will
be (2x + 3) and (2x + 5) respectively.
According to the given condition:
(2x + 1) + (2x + 3) + (2x + 5) = 51

⇒ 6x + 9 = 51 ∴ First number = 2x + 1 = 2 × 7 + 1 = 15,
⇒ 6x = 51 – 9 = 42 Second number = 2x + 3 = 2 × 7 + 3 = 17 and
Third number = 2x + 5 = 2 × 7 + 5 = 19.
⇒ x = 42 = 7
6
3
Example 6: Divide 400 into four parts, such that the second part is 5 of the first part, the third part is of the
6 6
first part and the fourth part is 1 of the first part. Find all the four parts.
3

5 3 1
Solution: It is given that second, third and fourth parts are , and of the first part respectively.
6 6 3
Let the first part be x. Then according to the given condition:

400 x 5 x 3 x 1 x ∴ First part = 150;
6 6 3 5
Second part = 150 125 ;
⇒ 400 × 6 = 6x + 5x + 3x + 2x [L.C.M. of 6, 6 and 3 is 6] 6
3
⇒ 2400 = 16x Third part 150 75 ;
6
1

⇒ x = 2400 =150 and Fourth part 150 50.
16 3

Example 7: A is 10 years older than B. The sum of their ages is 50. What are the ages of A and B?
Solution: It is given that A is 10 years older than B, and the sum of their ages is 50 years.

Let the present age of B be x years. Then, the present age of A will be (x + 10) years.
According to the given condition:
x + (x + 10) = 50

⇒ 2x + 10 = 50
⇒ 2x = 50 – 10 = 40 Hence, age of A = x + 10 = 20 + 10 = 30 years,

∴ x = 40 = 20 years and age of B = x = 20 years.
2

Example 8: Rajesh is 8 years older than Sailesh. In next 15 years, Rajesh will be twice as old as Sailesh was
15 years ago. Find their present ages.
Solution: Let the Sailesh’s present age be x years. Then, Rajesh’s present age will be (x + 8) years. Since in
15 years Rajesh will be twice as old as Sailesh was 15 years ago.

∴ x + 8 + 15 = 2 × (x – 15)